Steps is for finding the required steel reinforcements of beam with known M_max and other beam properties using Working Stress Design method.

Given the following, direct or indirect:

Consider a relatively long simply supported beam shown below. Assume the load w_o to be increasing progressively until the beam fails. The beam will go into the following three stages:

1. Uncrack Concrete Stage – at this stage, the gross section of the concrete will resist the bending which means that the beam will behave like a solid beam made entirely of concrete.
2. Crack Concrete Stage – Elastic Stress range
3. Ultimate Stress Stage – Beam Failure

Consistency is the term used to describe the ability of the soil to resist rupture and deformation. It is commonly describe as soft, stiff or firm, and hard.
 

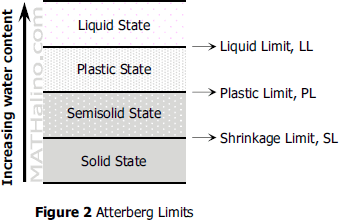
Water content greatly affects the engineering behavior of fine-grained soils. In the order of increasing moisture content (see Figure 2 below), a dry soil will exist into four distinct states: from solid state, to semisolid state, to plastic state, and to liquid state. The water contents at the boundary of these states are known as Atterberg limits. Between the solid and semisolid states is shrinkage limit, between semisolid and plastic states is plastic limit, and between plastic and liquid states is liquid limit.
 

Atterberg limits, then, are water contents at critical stages of soil behavior. They, together with natural water content, are essential descriptions of fine-grained soils.
 

Phase Diagram of Soil
Soil is composed of solids, liquids, and gases. Liquids and gases are mostly water and air, respectively. These two (water and air) are called voids which occupy between soil particles. The figure shown below is an idealized soil drawn into phases of solids, water, and air.
 

Weight-Volume Relationship from the Phase Diagram of Soil
total volume = volume of soilds + volume of voids
$V = V_s + V_v$

volume of voids = volume of water + volume of air
$V_v = V_w + V_a$

total weight = weight of solids + weight of water
$W = W_s + W_w$
 

Situation
Three reservoirs A, B, and C are connected respectively with pipes 1, 2, and 3 joining at a common junction P. Reservoir A is at elevation 80 m, reservoir B at elevation 70 m and reservoir C is at elevation 60 m. The properties of each pipe are as follows:

The flow from reservoir A to junction P is 0.045 m³/s and f for all pipes is 0.018.
 

1. Find the elevation of the energy grade line at P in m.
   A.   75.512
   B.   73.805
   C.   72.021
   D.   74.173
2. Determine the flow on pipe 2 in m³/s.
   A.   0.025
   B.   0.031
   C.   0.029
   D.   0.036
3. Compute the diameter appropriate for pipe 3 in mm.
   A.   175
   B.   170
   C.   178
   D.   172

Problem 20
The 600-mm pipe shown in Figure 4-11 conducts water from reservoir A to a pressure turbine, which discharges through another 600-mm pipe into tailrace B. The loss of head from A to 1 is 5 times the velocity head in the pipe and the loss of head from 2 to B is 0.2 times the velocity head in the pipe. If the discharge is 700 L/s, what power is being given up by the water to the turbine and what are the pressure heads at 1 and 2?
 

Problem 19
A pump draws water from reservoir A and lifts it to reservoir B as shown in Figure 4-10. The loss of head from A to 1 is 3 times the velocity head in the 150-mm pipe and the loss of head from 2 to B is 20 times the velocity head in the 100-mm pipe. Compute the horsepower output of the pump and the pressure heads at 1 and 2 when the discharge is: (a) 12 L/s; (b) 36 L/s.
 

Problem 18
Figure 4-09 shows a siphon discharging oil (sp gr 0.90). The siphon is composed of 3-in. pipe from A to B followed by 4-in. pipe from B to the open discharge at C. The head losses are from 1 to 2, 1.1 ft; from 2 to 3, 0.7 ft; from 3 to 4, 2.5 ft. Compute the discharge, and make table of heads at point 1, 2, 3, and 4.
 

Problem 17
In Figure 4-08 is shown a siphon discharging water from reservoir A into the air at B. Distance 'a' is 1.8 m, 'b' is 6 m, and the diameter is 150 mm throughout. If there is a frictional loss of 1.5 m between A and the summit, and 1.5 m between the summit and B, what is the absolute pressure at the summit in kiloPascal? Also determine the rate of discharge in cubic meter per second and in gallons per minute.
 

Problem 16
A pump (Figure 4-07) takes water from a 200-mm suction pipe and delivers it to a 150-mm discharge pipe in which the velocity is 3.6 m/s. The pressure is -35 kPa at A in the suction pipe. The 150-mm pipe discharges horizontally into air at C. To what height h above B can the water be raised if B is 1.8 m above A and 20 hp is delivered to the pump? Assume that the pump operates at 70 percent efficiency and that the frictional loss in the pipe between A and C is 3 m.