Physical Properties of Soil
Phase Diagram of Soil
Soil is composed of solids, liquids, and gases. Liquids and gases are mostly water and air, respectively. These two (water and air) are called voids which occupy between soil particles. The figure shown below is an idealized soil drawn into phases of solids, water, and air.
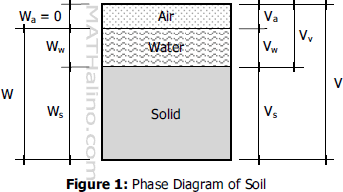
Weight-Volume Relationship from the Phase Diagram of Soil
total volume = volume of soilds + volume of voids
$V = V_s + V_v$
volume of voids = volume of water + volume of air
$V_v = V_w + V_a$
total weight = weight of solids + weight of water
$W = W_s + W_w$
Soil Properties
Void Ratio, e
Void ratio is the ratio of volume of voids to the volume of solids.
Porosity, n
Porosity is the ratio of volume of voids to the total volume of soil.
Degree of Saturation, S
Degree of saturation is the ratio of volume of water to the volume of voids.
Water Content or Moisture Content, w
Moisture content, usually expressed in terms of percentage, is the ratio of the weight of water to the weight of solids.
Unit Weight, γ
Unit weight is the weight of soil per unit volume. Also called bulk unit weight (γ), and moist unit weight (γm).
Dry Unit Weight, γd
Dry unit weight is the weight of dry soil per unit volume.
Saturated Unit Weight, γsat
Saturated unit weight is the weight of saturated soil per unit volume.
Effective Unit Weight, γ'
Effective unit weight is the weight of solids in a submerged soil per unit volume. Also called buoyant density or buoyant unit weight (γb).
Specific Gravity of Solid Particles, G
Specific gravity of solid particles of soil is the ratio of the unit weight of solids (γs) to the unit weight of water (γw).
Back to top
Formulas for Properties of Soil
Basic Formulas
Unit weight, $\gamma = s \gamma_w$
Weight, $W = \gamma V = s \gamma V$
Specific gravity, $s = \dfrac{\gamma}{\gamma_w}$
Physical Properties
Total weight, $W = W_w + W_s$
Total volume, $V = V_s + V_v$
Volume of voids, $V_v = V_w + V_a$
Void ratio, $e = \dfrac{V_v}{V_s}$, Note: $0 \lt e \lt \infty$
Porosity, $n = \dfrac{V_v}{V}$, Note: $0 \lt n \lt 1$
Relationship between e and n, $n = \dfrac{e}{1 + e}$ and $e = \dfrac{n}{1 - n}$
Water content or moisture content, $w = \dfrac{W_w}{W_s} \times 100\%$, Note: $0 \lt w \lt \infty$
Degree of saturation, $S = \dfrac{V_w}{V_v}$, Note: $0 \le S \le 1$
Relationship between G, w, S, and e, $Gw = Se$
Moist unit weight or bulk unit weight, $\gamma_m = \dfrac{W}{V} = \dfrac{(G + Se)\gamma_w}{1 + e} = \dfrac{G( 1 + w)\gamma_w}{1 + e}$
Dry unit weight, $\gamma_d = \dfrac{W_s}{V} = \dfrac{G\gamma_w}{1 + e}$
Saturated unit weight, $\gamma_{sat} = \dfrac{(G + e)\gamma_w}{1 + e}$
Submerged or buoyant unit weight, $\gamma_b = \gamma_{sat} - \gamma_w = \dfrac{(G - 1)\gamma_w}{1 + e}$
Critical hydraulic gradient, $i_{cr} = \dfrac{\gamma_b}{\gamma_w} = \dfrac{G - 1}{1 + e}$
Relative Density, $D_r = \dfrac{e_{max} - e}{e_{max} - e_{min}} = \dfrac{\dfrac{1}{(\gamma_d)_{min}} - \dfrac{1}{\gamma_d}}{\dfrac{1}{(\gamma_d)_{min}} - \dfrac{1}{(\gamma_d)_{max}}}$
Atterberg Limits
Plasticity index, $PI = LL - PL$
Liquidity index, $LI = \dfrac{MC - PL}{PI}$
Shrinkage index, $SI = PL - SL$
Activity of clay, $A_c = \dfrac{PI}{\mu}$, where $\mu$ = soil finer than 0.002 mm in percent
Other Formulas
Volume of voids, $V_v = \dfrac{eV}{1 + e}$
Volume of solids, $V_s = \dfrac{V}{1 + e}$
Volume of water, $V_w = \dfrac{SeV}{1 + e}$
Weight of water, $W_w = \dfrac{SeV \gamma_w}{1 + e}$
Weight of soil, $W = \dfrac{V(G + Se)\gamma_w}{1 + e}$
Dry unit weight, $\gamma_d = \dfrac{\gamma_m}{1 + w}$
Relationship between G, w, S, and e
The relationship between specific gravity of solid particles G, water content or moisture content w, degree of saturation S, and void ratio e, is given by the following:
The formula above can be derived as follows:
$\gamma_s = G\gamma_w$
$\dfrac{W_s}{V_s} = G\gamma_w$
$\dfrac{W_s}{V_s} \cdot \dfrac{W_w}{W_w} = G\gamma_w$
$\dfrac{W_w}{V_s} \cdot \dfrac{W_s}{W_w} = G\gamma_w$
$\dfrac{W_w}{V_s} \cdot \dfrac{1}{W_w/W_s} = G\gamma_w$
$\dfrac{\gamma_w V_w}{V_s} \cdot \dfrac{1}{w} = G\gamma_w$
$\dfrac{V_w}{V_s} \cdot \dfrac{1}{w} = G$
$\dfrac{V_w}{V_s} = Gw$
$\dfrac{V_w}{V_s} \cdot \dfrac{V_v}{V_v} = Gw$
$\dfrac{V_w}{V_v} \cdot \dfrac{V_v}{V_s} = Gw$
$Se = Gw$
Thus, $Gw = Se$ as stated above.
Relationship e and n
The relationship between the the void ratio e, and the porosity n is given by:
Derivation is as follows
$e = \dfrac{V_v}{V_s}$ ← void ratio
$e = \dfrac{V_v}{V - V_v}$
$e = \dfrac{V_v}{V - V_v} \cdot \dfrac{1/V}{1/V}$
$e = \dfrac{V_v/V}{1 - V_v/V}$ → n = Vv / V
$e = \dfrac{n}{1 - n}$ (okay!)
$n = \dfrac{V_v}{V}$ ← porosity
$n = \dfrac{V_v}{V_s + V_v}$
$n = \dfrac{V_v}{V_s + V_v} \cdot \dfrac{1/V_s}{1/V_s}$
$n = \dfrac{V_v/V_s}{1 + V_v/V_s}$ → e = Vv / Vs
$n = \dfrac{e}{1 + e}$ (okay!)
