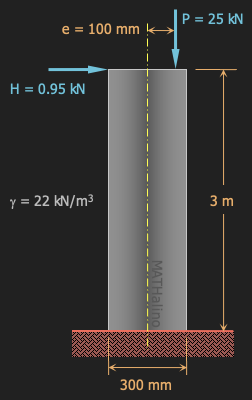
Ultimate Moment and Number of Bars of Simply Supported Concrete Beam
Situation
Given:
Effective Depth, d = 380 mm
Compressive Strength, fc' = 30 MPa
Steel Strength, fy = 415 MPa
- The beam is simply supported on a span of 5 m, and carries the following loads:
- Superimposed Dead Load = 16 kN/m
Live Load = 14 kN/mWhat is the maximum moment, Mu (kN·m), at ultimate condition? $U = 1.4D + 1.7L$
A. 144 C. 104 B. 158 D. 195 - Superimposed Dead Load = 16 kN/m
- Find the number of 16 mm diameter bars required if the design moment at ultimate loads is 200 kN·m.
A. 2 C. 6 B. 4 D. 8 - If the beam carries an ultimate concentrated load of 50 kN at midspan, what is the number of 16 mm diameter bars required?
A. 2 C. 4 B. 3 D. 5