Example 04: Required Depth of Rectangular Timber Beam Based on Allowable Bending, Shear, and Deflection

Problem
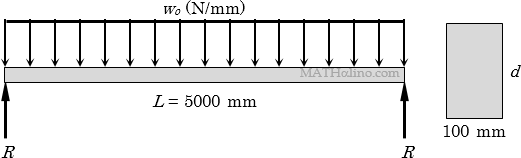
A beam 100 mm wide is to be loaded with 3 kN concentrated loads spaced uniformly at 0.40 m on centers throughout the 5 m span. The following data are given:

Allowable bending stress = 24 MPa
Allowable shear stress = 1.24 MPa
Allowable deflection = 1/240 of span
Modulus of elasticity = 18,600 MPa
Weight of wood = 8 kN/m3
  1. Find the depth d considering bending stress only.
  2. Determine the depth d considering shear stress only.
  3. Calculate the depth d considering deflection only.

 

beam-003-required-depth.gif

 

Example 03: Moment Capacity of a Timber Beam Reinforced with Steel and Aluminum Strips

Problem
Steel and aluminum plates are used to reinforced an 80 mm by 150 mm timber beam. The three materials are fastened firmly as shown so that there will be no relative movement between them.
 

beam-002-wood-reinforced-steel-aluminum.gif

 

Given the following material properties:

Allowable Bending Stress, Fb
Steel = 120 MPa
Aluminum = 80 MPa
Wood = 10 MPa		 Modulus of Elasticity, E
Steel = 200 GPa
Aluminum = 70 GPa
Wood = 10 GPa

Find the safe resisting moment of the beam in kN·m.
 

Example 02: Required Diameter of Circular Log Used for Footbridge Based on Shear Alone

Problem
A wooden log is to be used as a footbridge to span 3-m gap. The log is required to support a concentrated load of 30 kN at midspan. If the allowable stress in shear is 0.7 MPa, what is the diameter of the log that would be needed. Assume the log is very nearly circular and the bending stresses are adequately met. Neglect the weight of the log.
 

beam-001-circular-log-shear-stress.gif

 

Example 01: Maximum bending stress, shear stress, and deflection

Problem
A timber beam 4 m long is simply supported at both ends. It carries a uniform load of 10 kN/m including its own weight. The wooden section has a width of 200 mm and a depth of 260 mm and is made up of 80% grade Apitong. Use dressed dimension by reducing its dimensions by 10 mm.

Properties of Apitong
Bending and tension parallel to grain = 16.5 MPa
Shear parallel to grain = 1.73 MPa
Modulus of elasticity in bending = 7.31 GPa
  1. What is the maximum flexural stress of the beam?
  2. What is the maximum shearing stress of the beam?
  3. What is the maximum deflection of the beam?

 

2006-may-ce-board-stresses-in-timber-beam.gif

 

Safe Dimensions of the Notch at a Joint of a Timber Truss

Situation
The truss shown in is made from timber Guijo 100 mm × 150 mm. The load on the truss is 20 kN. Neglect friction.

Allowable stresses for Guijo:
Compression parallel to grain = 11 MPa
Compression perpendicular to grain = 5 MPa
Shear parallel to grain = 1 MPa

 

2015-may-design-timber-3member-truss-triangular.gif

 

1.   Determine the minimum value of x in mm.

A.   180 C.   160
B.   150 D.   140

2.   Determine the minimum value of y in mm.

A.   34.9 C.   13.2
B.   26.8 D.   19.5

3.   Calculate the axial stress of member AC in MPa.

A.   1.26 C.   1.57
B.   1.62 D.   1.75

 

Maximum Stress of Truss Member Due to Moving Loads

Situation
The bridge truss shown in the figure is to be subjected by uniform load of 10 kN/m and a point load of 30 kN, both are moving across the bottom chord
 

2014-may-design-truss-equilateral-triangle-given.gif

 

Calculate the following:
1.   The maximum axial load on member JK.

A.   64.59 kN C.   -64.59 kN
B.   -63.51 kN D.   63.51 kN

2.   The maximum axial load on member BC.

A.   47.63 kN C.   -47.63 kN
B.   -74.88 kN D.   74.88 kN

3.   The maximum compression force and maximum tension force on member CG.

A.   -48.11 kN and 16.36 kN
B.   Compression = 0; Tension = 16.36 kN
C.   -16.36 kN and 48.11 kN
D.   Compression = 48.11 kN; Tension = 0

 

Truss With Tension-Only Diagonals

Situation
Diagonals BG, CF, CH, and DG of the truss shown can resist tension only.
 

2016-may-design-truss-with-tension-diagonals.gif

 

If W = 3 kN and P = 0, find the following:
1.   the force in member CF.

A.   4.76 kN C.   4.67 kN
B.   4.32 kN D.   4.23 kN

2.   the force in member BF.

A.   3.2 kN C.   3.4 kN
B.   3.3 kN D.   3.5 kN

3.   the force in member DH.

A.   2.8 kN A.   2.5 kN
B.   2.8 kN D.   2.7 kN

 

3-Panel Truss with Flexible Cables Used as Diagonals

Situation
Flexible cables BE and CD are used to brace the truss shown below.
 

2016-may-design-3panel-truss-counter-diagonals.gif

 

1.   Determine the load W to cause a compression force of 8.9 kN to member BD.

A.   7.80 kN C.   26.70 kN
B.   35.64 kN D.   13.35 kN

2.   Which cable is in tension and what is the tensile reaction?

A.   BE = 12.58 kN C.   BE = 6.29 kN
B.   CD = 6.29 kN D.   CD = 12.58 kN

3.   If W = 20 kN, what will be the tensile reaction of member CE?

A.   6.67 kN C.   0
B.   13.33 kN D.   10 kN