$\Sigma M_D = 0$
$2xR_A = 450x$
$R_A = 225 \, \text{N}$
$\Sigma �M_A = 0$
$2xV_D = 450x + (450 \sin 30^\circ)(2x)$
$V_D = 450 \, \text{N}$
$\Sigma �F_H = 0$
$H_D = 450 \cos 30^\circ = 389.71 \, \text{N}$
 At Joint A
At Joint A
�$\Sigma F_V = 0$
$F_{AB} \sin 30^\circ = 225$
$F_{AB} = 450 \, \text{N}$
$\Sigma �F_H = 0$
$F_{AC} = F_{AB} \cos 30^\circ = 450 \cos 30^\circ$
$F_{AC} = 389.71 \, \text{N}$
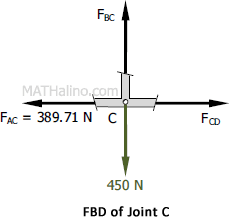 At Joint C
At Joint C
$\Sigma �F_V = 0$
$F_{BC} = 450 \, \text{N}$
$\Sigma �F_H = 0$
$F_{CD} = 389.71 \, \text{N}$
At Joint B
�$\Sigma F_H = 0$
$F_{BD} \cos 30^\circ = 450 \cos 30^\circ + 450 \cos 30^\circ$
$F_{BD} = 900 \, \text{N}$
$\Sigma �F_V = 0$
$F_{BD} \sin 30^\circ + 450 \sin 30^\circ = 450 + 450 \sin 30^\circ$
$F_{BD} = 900 \, \text{N}$ Check!
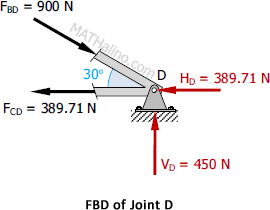 At Joint D
At Joint D
$\Sigma �F_V = 0$
$450 = 900 \sin 30^\circ$
$450 = 450$ Check!
$\Sigma �F_H = 0$
$900 \cos 30^\circ = 389.71 + 389.71$
$779.42 = 779.42$ Check!
Summary
AB = 450 N compression
AC = 389.71 N tension
BC = 450 N tension
BD = 900 N compression
CD = 389.71 N tension

In the solution, second line
In the solution, second line under the first sketch (of reaction forces at supports):
the sum of the moments about A = 2xVD=450x+(450sin30∘)(2x)
How is the last term (2x) determined?
The 450 N at B was resolved
In reply to In the solution, second line by daveB
The 450 N at B was resolved into components at D. The vertical downward component is at distance 2x from A.
I tried solving this part but
In reply to In the solution, second line by daveB
I tried solving this part but this time, considering all forces with respect to point A, here's what I got:
VD(2x) = 450(x)+(450sin30)(x)+(450cos30)(xtan30)
VD=450
I think what they've shown is a simplified equation already.