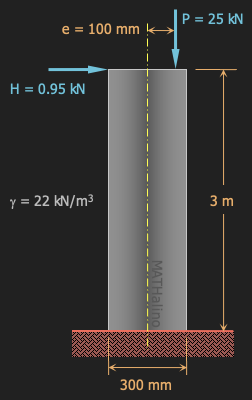
Equivalent land area of 600 mm^2 map-area with given map-scale
Problem
The area of a park on a map is 600 mm2. If the scale of the map is 1 to 40,000 determine the true area of the park in hectares (1 hectare = 104 m2).
| A. 112 | C. 96 |
| B. 84 | D. 120 |
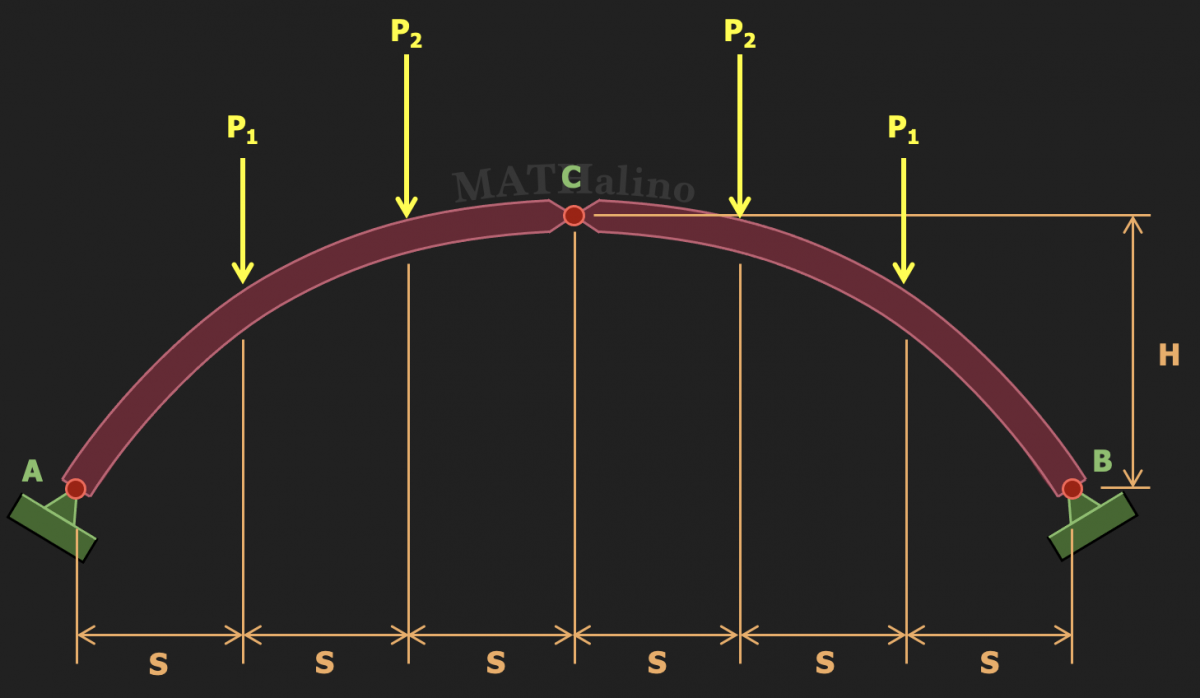
Support reactions of a symmetrically-loaded three-hinged arch structure
Situation
The three-hinged arch shown below is loaded with symmetrically placed concentrated loads as shown in the figure below.
The loads are as follows:
$$P_1 = 90 ~ \text{kN} \qquad P_2 = 240 ~ \text{kN}$$
The dimensions are:
$$H = 8 ~ \text{m} \qquad S = 4 ~ \text{kN}$$
Calculate the following:
1. The horizontal reaction at A.
| A. 0 | C. 330 kN |
| B. 285 kN | D. 436 kN |
2. The total reaction at B.
| A. 0 | C. 330 kN |
| B. 285 kN | D. 436 kN |
3. The vertical reaction at C.
| A. 0 | C. 330 kN |
| B. 285 kN | D. 436 kN |
Evaluate the integral of (x dx) / (x^2 + 2) with lower limit of 0 and upper limit of 1
Problem
Evaluate $\displaystyle \int_0^1 \dfrac{x \, dx}{x^2 + 2}$.
| A. 0.2027 | C. 0.2270 |
| B. 0.2207 | D. 0.2072 |
Determine the radius of curvature of the curve x = y^3 at point (1, 1)
Problem
Determine the radius of curvature of the curve $x = y^3$ at point (1, 1).
| A. 5.27 | C. 5.56 |
| B. 5.65 | D. 5.72 |
Calculate the area enclosed by the curve x^2 + y^2 - 10x + 4y - 196 = 0.
Problem
Calculate the area enclosed by the curve $x^2 + y^2 - 10x + 4y - 196 = 0$.
| A. 15π | C. 169π |
| B. 13π | D. 225π |
Sum of the first ten terms of a Geometric Progression
Problem
The first three terms of a geometric progression are 2x, 4x + 14 and 20x - 14. Find the sum of the first ten terms.
| A. 413,633 | C. 489,335 |
| B. 498,533 | D. 431,336 |
- Read more about Sum of the first ten terms of a Geometric Progression
- Log in or register to post comments
Calculation of true distance of a line measuring 160.42 m using a tape that is 0.02m too long
Problem
A 50-m steel tape that is is 0.02 m too long was used to measure the distance between two points A and B. If the measured distance was 160.42 m, calculate the correct distance between A and B.
| A. 160.356 m | C. 160.844 m |
| B. 160.484 m | D. 160.563 m |
A circle has an equation of x^2 + y^2 + 2cy = 0. Find the value of c when the length of the tangent from (5, 4) to the circle is equal to one.
Problem
A circle has an equation of $x^2 + y^2 + 2cy = 0$. Find the value of $c$ when the length of the tangent from (5, 4) to the circle is equal to one.
| A. 5 | C. 3 |
| B. -3 | D. -5 |
Calculate the acute angle between 2x - 4y - z = -5 and 3x + 4y + 5z = -6
Problem
Calculate the acute angle between two intersecting surfaces whose equations are as follows:
$$2x - 4y - z = -5$$
$$3x + 4y + 5z = -6$$
| A. 62.4° | C. 42.6° |
| B. 64.2° | D. 46.2° |

Recent comments
(…