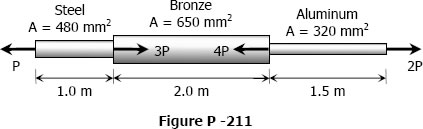
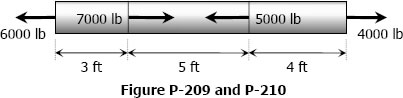
Solution to Problem 217 Axial Deformation
Problem 217
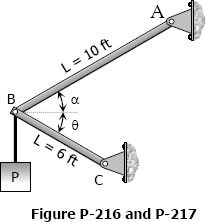
Solve Prob. 216 if rod AB is of steel, with E = 29 × 106 psi. Assume α = 45° and θ = 30°; all other data remain unchanged.
- Read more about Solution to Problem 217 Axial Deformation
- Log in or register to post comments


Recent comments
(…