Frustum of a right circular cone is that portion of right circular cone included between the base and a section parallel to the base not passing through the vertex.
 

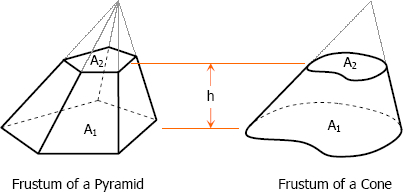
Frustum of a regular pyramid is a portion of right regular pyramid included between the base and a section parallel to the base.
 

Frustum of a pyramid (or cone) is a portion of pyramid (or cone) included between the base and the section parallel to the base not passing through the vertex.
 

Cone
The surface generated by a moving straight line (generator) which always passes through a fixed point (vertex) and always intersects a fixed plane curve (directrix) is called conical surface. Cone is a solid bounded by a conical surface whose directrix is a closed curve, and a plane which cuts all the elements. The conical surface is the lateral area of the cone and the plane which cuts all the elements is the base of the cone.
 

Two surfaces or solids are similar if any two corresponding sides or planes are proportional.
 

Problem 670
Determine the value of EIδ at the left end of the overhanging beam shown in Fig. P-670.
 

Problem 669
Compute the value of EIδ midway between the supports of the beam shown in Fig. P-669.
 

Problem 668
For the beam shown in Fig. P-668, compute the value of P that will cause the tangent to the elastic curve over support R₂ to be horizontal. What will then be the value of EIδ under the 100-lb load?
 

Problem 667
Determine the value of EIδ at the right end of the overhanging beam shown in Fig. P-667. Is the deflection up or down?