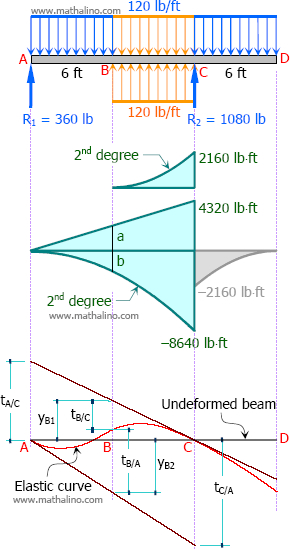
 ΣMR2=0
ΣMR2=0
12R1+3(6)(120)=9(6)(120)
R1=360 lb
ΣMR1=0
12R2=3(6)(120)+15(6)(120)
R2=1080 lb
By ratio and proportion:
a6=432012
a=2160 lb⋅ft
By squared property of parabola:
b62=−8640122
b=−2160 lb⋅ft
EItC/A=(AreaAC)ˉXC
EItC/A=13(6)(2160)(32)+12(12)(4320)(4)−13(12)(8640)(3)
EItC/A=6480 lb⋅ft3
EItB/A=(AreaAB)ˉXB
EItB/A=12(6a)(2)−13(6b)(32)
EItB/A=6a−3b
EItB/A=6(2160)−3(2160)
EItB/A=6480 lb⋅ft3
With the values of EI tC/A and EI tB/A, it is obvious that the elastic curve is above point B. The deflection at B (up or down) can also be determined by comparing the values of tB/A and yB2.
By ratio and proportion:
yB26=tC/A12
yB2=12tC/A
EIyB2=12EItC/A
EIyB2=12(6480)
EIyB2=3240 lb⋅ft3
Since tB/A is greater than yB2, the elastic curve is above point B as concluded previously.
Therefore,
EIδB=EItB/A−EIyB2
EIδB=6480−3240
EIδB=3240 lb⋅ft3 answer
You can also find the value EI δB by finding tA/C, tB/C, and yB1. I encourage you to do it yourself.
