The Cone

Cone
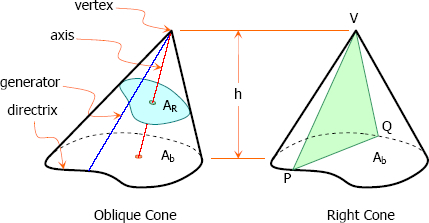
The surface generated by a moving straight line (generator) which always passes through a fixed point (vertex) and always intersects a fixed plane curve (directrix) is called conical surface. Cone is a solid bounded by a conical surface whose directrix is a closed curve, and a plane which cuts all the elements. The conical surface is the lateral area of the cone and the plane which cuts all the elements is the base of the cone.
 

Like pyramids, cones are generally classified according to their bases.
 

Volume of a Cone

Properties of a Cone

• An element of a cone is the generator in any particular position.
• The altitude of the cone is the perpendicular drop from vertex to the plane of the base. It is denoted as h.
• Every section of a cone made by a plane passing through its vertex and containing two points of the base is a triangle. See section PQV, where V is the vertex and P and Q are two points on the base.
• The axis of the cone is the straight line joining the vertex with the centroid of the base. For right cone, altitude and axis are equal in length.
• The right section of a cone is a section perpendicular to its axis and cutting all the elements. For right cone, the right section is parallel and similar to the base. Right section is denoted by A_R.
• A circular cone is cone whose right section is a circle.