The Right Circular Cylinder
A right circular cylinder is a cylinder whose base is a circle and whose elements are perpendicular to its base.
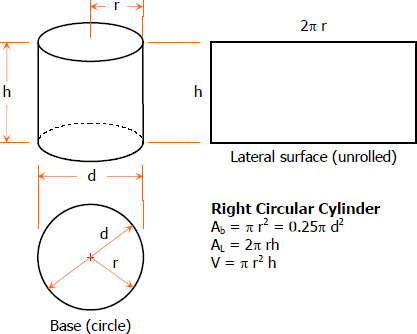
Properties of a Right Circular Cylinder
- The axis of a right circular cylinder is the line joining the centers of the bases.
- For any oblique or non-oblique sections which do not pass any one base, the center of which is at the axis.
- A right circular cylinder can be formed by revolving a rectangle about one side as axis of revolution.
- Every section of a right circular cylinder made by a cutting plane containing two elements and parallel to the axis is a rectangle.
Formulas for Right Circular Cylinder
$A_b = \pi r^2$
$A_b = \dfrac{\pi}{4}d^2$
$A_L = 2\pi \, rh$
$A_L = \pi \, dh$
$V = A_b h$
$V = \pi r^2 h$
$V = \dfrac{\pi}{4} d^2 h$
Total area (open both ends), $A_T = A_L$
Total Area (open one end), $A_T = A_b + A_L$
Total Area (closed both ends), $A_T = 2A_b + A_L$
