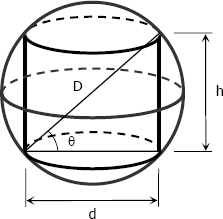
A shot is in the form of a sphere and the cylinder is the cylinder of maximum. From the figure )This is also the
figure used in Solution 02:
Vc=14πd2h
Where:
d=Dcosθ
h=Dsinθ
Thus,
Vc=14π(Dcosθ)2(sinθ)
Vc=14D3πcos2θsinθ
dVcdθ=14D3π[cos2θ(cosθ)+sinθ(−2cosθsinθ)]
dVcdθ=14D3π(cos3θ−2sin2θcosθ)=0
2sin2θcosθ=cos3θ
2sin2θ=cos2θ
sin2θcos2θ=12
tan2θ=12
tanθ=1√2
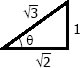 cosθ=√2√3
cosθ=√2√3
sinθ=1√3
Vc=14D3π(√2√3)2(1√3)
Vc=16√3πD3 → Maximum volume of cylinder
Volume of shot (sphere):
Vs=43πR3=43π(D/2)3
Vs=16πD3
Weight is proportional to the volume, so
WcVc=WsVs
Wc=WsVs×Vc
Wc=1616πD3×16√3πD3
Wc=9.24 lb answer
