Solution to Problem 692 | Beam Deflection by Method of Superposition

The midspan deflection from Case No. 8 and Case No. 11 are respectively,
 

$\delta = \dfrac{5w_oL^4}{384EI}$
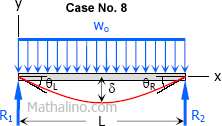$\delta = \dfrac{ML^2}{16EI}$

 

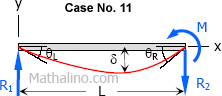The given beam is transformed into a simple beam with end moment at the right support due to the load at the overhang as shown in the figure below.
 

 

EIδ = ½ of EIδ due to uniform load over the entire span - EIδ due to end moment

$EI \, \delta_{midspan} = \dfrac{1}{2}\left[ \dfrac{5(120)(12^4}{384} \right] - \dfrac{2160(12^2)}{16}$

$EI \, \delta_{midspan} = 16\,200 - 19\,440$

$EI \, \delta_{midspan} = -3\,240$

$EI \, \delta_{midspan} = 3\,240 \, \text{ lb}\cdot\text{ft}^3 \, \text{ upward}$           answer