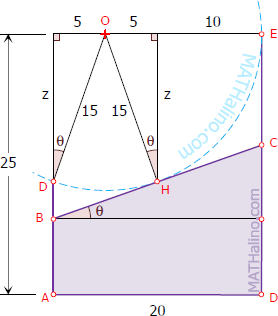
As described by
Alexander Bogomolny of cut-the-knot.org, for maximum area of trapezoid, the point of tangency should be at the midline of AB and DC, thus H is the midpoint of BC.
From the figure:
$z^2 + 5^2 = 15^2$
$z = 10\sqrt{2}$
For the angle theta:
$\cos \theta = \dfrac{z}{15} = \dfrac{20}{BC}$
$BC = \dfrac{300}{z} = \dfrac{300}{10\sqrt{2}}$
$BC = 15\sqrt{2} \, \text{ cm} = 21.21 \, \text{ cm}$ answer
A solution to the same problem has been carried out with the aid of Calculus. See this link to to see the solution by maxima and minima: Solution by Calculus
