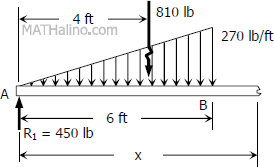
$\Sigma M_C = 0$
$9R_1 = 5(810)$
$R_1 = 450 \, \text{lb}$
$\Sigma M_A = 0$
$9R_2 = 4(810)$
$R_2 = 360 \, \text{lb}$
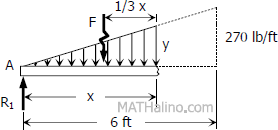 Segment AB:
Segment AB:
$\dfrac{y}{x} = \dfrac{270}{6}$
$y = 45x$
$F = \frac{1}{2}xy = \frac{1}{2}x(45x)$
$F = 22.5x^2$
$V_{AB} = R_1 - F$
$V_{AB} = 450 - 22.5x^2 \, \text{lb}$
$M_{AB} = R_1x - F(\frac{1}{3}x)$
$M_{AB} = 450x - 22.5x^2(\frac{1}{3}x)$
$M_{AB} = 450x - 7.5x^3 \, \text{lb}\cdot\text{ft}$
 Segment BC:
Segment BC:
$V_{BC} = 450 - 810$
$V_{BC} = -360 \, \text{lb}$
$M_{BC} = 450x - 810(x - 4)$
$M_{BC} = 450x - 810x + 3240$
$M_{BC} = 3240 - 360x \, \text{lb}\cdot\text{ft}$
To draw the Shear Diagram:
- VAB = 450 - 22.5x2 is a second degree curve; at x = 0, VAB = 450 lb; at x = 6 ft, VAB = -360 lb.
- At x = a, VAB = 0,
450 - 22.5x2 = 0
22.5x2 = 450
x2 = 20
x = √20
To check, use the squared property of parabola.
a2/450 = 62/(450 + 360)
a2 = 20
a = √20
- VBC = -360 lb is constant.
To draw the Moment Diagram:
- MAB = 450x - 7.5x3 for segment AB is third degree curve; at x = 0, MAB = 0; at x = √20, MAB = 1341.64 lb·ft; at x = 6 ft, MAB = 1080 lb·ft.
- MBC = 3240 - 360x for segment BC is linear; at x = 6 ft, MBC = 1080 lb·ft; at x = 9 ft, MBC = 0.
