$\Sigma M_C = 0$
$2R(R_A) = RP$
$R_A = \frac{1}{2} P$
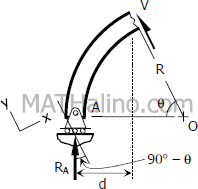
For θ that is less than 90°
Shear:
$V_{AB} = R_A \cos (90^\circ - \theta)$
$V_{AB} = \frac{1}{2}P \, (\cos 90^\circ \cos \theta + \sin 90^\circ \sin \theta)$
$V_{AB} = \frac{1}{2}P \sin \theta$ answer
Moment arm:
$d = R - R \cos \theta$
$d = R(1 - \cos \theta)$
Moment:
$M_{AB} = R_A (d)$
$M_{AB} = \frac{1}{2}PR \, (1 - \cos \theta)$ answer
For θ that is greater than 90°
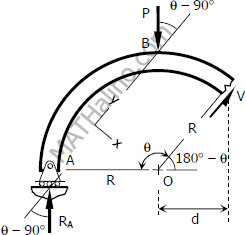Components of P and RA:
$P_x = P \sin (\theta - 90^\circ)$
$P_x = P (\sin \theta \cos 90^\circ - \cos \theta \sin 90^\circ)$
$P_x = -P \cos \theta$
$P_y = P \cos (\theta - 90^\circ)$
$P_y = P (\cos \theta \cos 90^\circ + \sin \theta \sin 90^\circ)$
$P_y = P \sin \theta$
$R_{Ax} = R_A \sin (\theta - 90^\circ)$
$R_{Ax} = \frac{1}{2}P (\sin \theta \cos 90^\circ - \cos \theta \sin 90^\circ)$
$R_{Ax} = -\frac{1}{2}P \cos \theta$
$R_{Ay} = R_A \cos (\theta - 90^\circ)$
$R_{Ay} = \frac{1}{2}P (\cos \theta \cos 90^\circ + \sin \theta \sin 90^\circ)$
$R_{Ay} = \frac{1}{2}P \sin \theta$
Shear:
$V_{BC} = \Sigma F_y$
$V_{BC} = R_{Ay} - P_y$
$V_{BC} = \frac{1}{2}P \sin \theta - P \sin \theta$
$V_{BC} = -\frac{1}{2}P \sin \theta$ answer
Moment arm:
$d = R \cos (180^\circ - \theta)$
$d = R (\cos 180^\circ \cos \theta + \sin 180^\circ \sin \theta)$
$d = -R \cos \theta$
Moment:
$M_{BC} = \Sigma M_{counterclockwise} - \Sigma M_{clockwise}$
$M_{BC} = R_A(R + d) - Pd$
$M_{BC} = \frac{1}{2}P(R - R \cos \theta) - P(-R \cos \theta)$
$M_{BC} = \frac{1}{2}PR - \frac{1}{2}PR \cos \theta + PR \cos \theta$
$M_{BC} = \frac{1}{2}PR + \frac{1}{2}PR \cos \theta$
$M_{BC} = \frac{1}{2}PR\,(1 + \cos \theta)$ answer
