Moving Loads
Moving Loads
From the previous section, we see that the maximum moment occurs at a point of zero shears. For beams loaded with concentrated loads, the point of zero shears usually occurs under a concentrated load and so the maximum moment.
Beams and girders such as in a bridge or an overhead crane are subject to moving concentrated loads, which are at fixed distance with each other. The problem here is to determine the moment under each load when each load is in a position to cause a maximum moment. The largest value of these moments governs the design of the beam.
Single Moving Load
For a single moving load, the maximum moment occurs when the load is at the midspan and the maximum shear occurs when the load is very near the support (usually assumed to lie over the support).
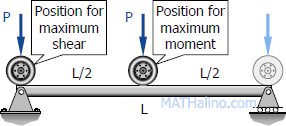
Two Moving Loads
For two moving loads, the maximum shear occurs at the reaction when the larger load is over that support. The maximum moment is given by

where Ps is the smaller load, Pb is the bigger load, and P is the total load (P = Ps + Pb).
Three or more moving loads
In general, the bending moment under a particular load is a maximum when the center of the beam is midway between that load and the resultant of all the loads then on the span. With this rule, we compute the maximum moment under each load, and use the biggest of the moments for the design. Usually, the biggest of these moments occurs under the biggest load.
The maximum shear occurs at the reaction where the resultant load is nearest. Usually, it happens if the biggest load is over that support and as many a possible of the remaining loads are still on the span.
In determining the largest moment and shear, it is sometimes necessary to check the condition when the bigger loads are on the span and the rest of the smaller loads are outside.
