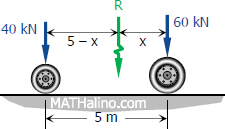
$R = 40 + 60 = 100 \, \text{kN}$
$xR = 40(5)$
$x = 200/R$
$x = 200/100$
$x = 2 \, \text{m}$
For maximum moment under 40 kN wheel:
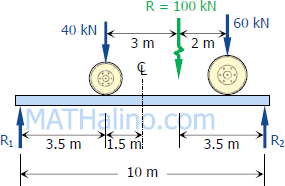$\Sigma M_{R2} = 0$
$10R_1 = 3.5(100)$
$R_1 = 35 \, \text{kN}$
$M_{To\,\,the\,\,left\,\,of\,\,40\,\,kN} = 3.5R_1$
$M_{To\,\,the\,\,left\,\,of\,\,40\,\,kN} = 3.5(35)$
$M_{To\,\,the\,\,left\,\,of\,\,40\,\,kN} = 122.5 \, \text{kN}\cdot\text{m}$
For maximum moment under 60 kN wheel:
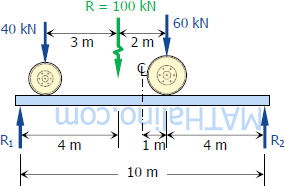$\Sigma M_{R1} = 0$
$10R_2 = 4(100)$
$R_2 = 40 \, \text{kN}$
$M_{To\,\,the\,\,right\,\,of\,\,60\,\,kN} = 4R_2$
$M_{To\,\,the\,\,right\,\,of\,\,60\,\,kN} = 4(40)$
$M_{To\,\,the\,\,right\,\,of\,\,60\,\,kN} = 160 \, \text{kN}\cdot\text{m}$
Thus, $M_{max} = 160 \, \text{kN}\cdot\text{m} \,\, $ answer
The maximum shear will occur when the 60 kN is over a support.
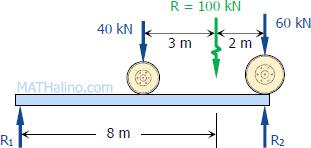$\Sigma M_{R1} = 0$
$10R_2 = 100(8)$
$R_2 = 80 \, \text{kN}$
Thus, $V_{max} = 80 \, \text{kN} \,\, $ answer
