Solution to Problem 411 | Shear and Moment Diagrams

$y = \dfrac{w_o}{L}(L - x)$
 

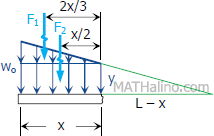$F_1 = \frac{1}{2}x(w_o - y)$

$F_1 = \dfrac{1}{2}x \, \left[ w_o - \dfrac{w_o}{L}(L - x) \right]$

$F_1 = \dfrac{1}{2}x \, \left[ w_o - w_o + \dfrac{w_o}{L}x \right]$

$F_1 = \dfrac{w_o}{2L}x^2$
 

$F_2 = xy = x \, \left[ \dfrac{w_o}{L}(L - x) \right]$

$F_2 = \dfrac{w_o}{L}(Lx - x^2)$
 

Shear equation:
$V = -F_1 - F_2 = -\dfrac{w_o}{2L}x^2 - \dfrac{w_o}{L}(Lx - x^2)$

$V = -\dfrac{w_o}{2L}x^2 - w_ox + \dfrac{w_o}{L}x^2$

$V = \dfrac{w_o}{2L}x^2 - w_ox$
 

Moment equation:
$M = -\frac{2}{3}x F_1 - \frac{1}{2}x F_2$

$M = -\dfrac{2}{3}x \, \left( \dfrac{w_o}{2L}x^2 \right) - \frac{1}{2}x \, \left[ \dfrac{w_o}{L}(Lx - x^2) \right]$

$M = -\dfrac{w_o}{3L}x^3 - \dfrac{w_o}{2}x^2 + \dfrac{w_o}{2L}x^3$

$M = -\dfrac{w_o}{2}x^2 + \dfrac{w_o}{6L}x^3$
 

To draw the Shear Diagram:

1. V = w_ox²/2L - w_ox is a concave upward second degree curve; at x = 0, V = 0; at x = L, V = -1/2 w_oL.

To draw the Moment diagram:

1. M = -w_ox²/2 + w_ox³/6L is in third degree; at x = 0, M = 0; at x = L, M = -1/3 w_oL².