Derivation of Pythagorean Theorem

Pythagorean Theorem
In any right triangle, the sum of the square of the two perpendicular sides is equal to the square of the longest side. For a right triangle with legs measures $a$ and $b$ and length of hypotenuse $c$, the theorem can be expressed in the form
 

 

Proved by Pythagoras
 

 

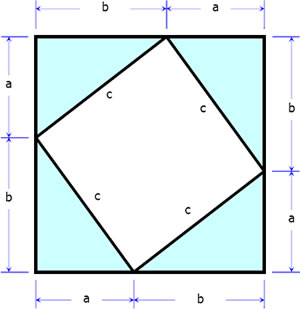
Area of the large square = Area of four triangles + Area of small square
$A_{total} = A_{four \, \, triangles} + A_{small \, \, square}$

$(a + b)^2 = 4 \, \left( \frac{1}{2} ab \right) + c^2$

$a^2 + 2ab + b^2 = 2ab + c^2$

$a^2 + b^2 = c^2$
 

Proved by Bhaskara
Bhaskara (1114 - 1185) was an Indian mathematician and astronomer.
 

 

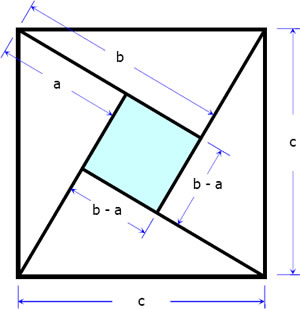
Area of the large square = Area of four triangles + Area of inner (smaller) square
$A_{total} = A_{four \, \, triangles} + A_{small \, \, square}$

$c^2 = 4 \, \left( \frac{1}{2} ab \right) + (b - a)^2$

$c^2 = 2ab + (b^2 - 2ab + a^2)$

$c^2 = 2ab + b^2 - 2ab + a^2$

$c^2 = b^2 + a^2$
 

Proved by U.S. Pres. James Garfield
 

 

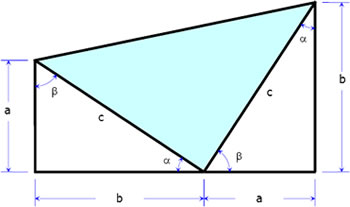
Area of trapezoid = Area of 3 triangles
$\frac{1}{2}(a + b)(a + b) = \frac{1}{2}ab + \frac{1}{2}c^2 + \frac{1}{2}ab$

$(a + b)^2 = ab + c^2 + ab$

$a^2 + 2ab + b^2 = 2ab + c^2$

$a^2 + b^2 = c^2$