Derivation of Basic Identities

Tags: 
identity
trigonometric identities

The derivation of basic identities can be done easily by using the functions of a right triangle. For easy reference, these trigonometric functions are listed below.
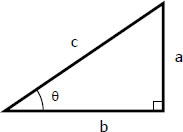Right triangle with sides a, b, and c and angle theta

a/c = sin θ
b/c = cos θ
a/b = tan θ
c/a = csc θ
c/b = sec θ
b/a = cot θ

 

Sine and Cosecant are reciprocal to each other
$ \sin \theta = \dfrac{a}{c} $

$ \sin \theta = \dfrac{a/a}{c/a} $

$ \sin \theta = \dfrac{1}{\csc \theta} $

and

$ \csc \theta = \dfrac{1}{\sin \theta} $

 

Cosine and Secant are reciprocal to each other
$ \cos \theta = \dfrac{b}{c} $

$ \cos \theta = \dfrac{b/b}{c/b} $

$ \cos \theta = \dfrac{1}{\sec \theta} $

and

$ \sec \theta = \dfrac{1}{\cos \theta} $

 

Tangent and Cotangent are reciprocal to each other and Tangent is the ratio of Sine to Cosine
$ \tan \theta = \dfrac{a}{b} $

$ \tan \theta = \dfrac{a/a}{b/a} $

$ \tan \theta = \dfrac{1}{\cot \theta} $
 

$ \tan \theta = \dfrac{a}{b} $

$ \tan \theta = \dfrac{a/c}{b/c} $

$ \tan \theta = \dfrac{\sin \theta}{\cos \theta} $
 

Thus,

$ \tan \theta = \dfrac{\sin \theta}{\cos \theta} = \dfrac{1}{\cot \theta} $

and

$ \cot \theta = \dfrac{\cos \theta}{\sin \theta} = \dfrac{1}{\tan \theta} $

 

»

Comments

Submitted by Kimberly Mansalayao on April 17, 2013 - 2:36pm

One can always reason with reason.

SPONSORED LINKS


Page copy protected against web site content infringement by Copyscape

Recent comments