Plane Areas in Rectangular Coordinates | Applications of Integration

There are two methods for finding the area bounded by curves in rectangular coordinates. These are...

1. by using a horizontal element (called strip) of area, and
2. by using a vertical strip of area.

The strip is in the form of a rectangle with area equal to length × width, with width equal to the differential element. To find the total area enclosed by specified curves, it is necessary to sum up a series of rectangles defined by the strip.
 

Using Horizontal Strip

 
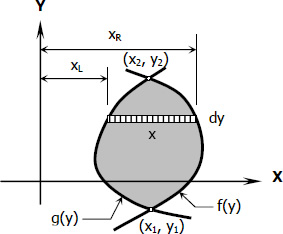
From the figure, the area of the strip is $x \, dy$, where $x = x_R - x_L$. The total area can be found by running this strip starting from $y_1$ going to $y_2$. Our formula for integration is...
 

 
Note that $x_R$ is the right end of the strip and is always on the curve $f(y)$ and $x_L$ is the left end of the strip and is always on the curve $g(y)$. We therefore substitute $x_R = f(y)$ and $x_L = g(y)$ prior to integration.
 

Using Vertical Strip

We apply the same principle of using horizontal strip to the vertical strip. Consider the figure below.
 

 
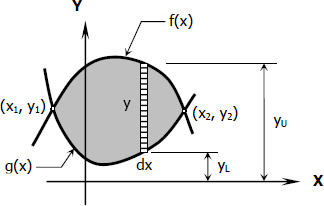
The total area is...
 

 
Where
$y_U$ = upper end of the strip = $f(x)$
$y_L$ = lower end of the strip = $g(x)$
 
The steps in finding the area can be outlined as follows:
1. Sketch the curve
2. Decide what strip to use and define its limits
3. Apply the appropriate formula based on the strip then integrate.
 
Browse for some examples that illustrate the two methods.