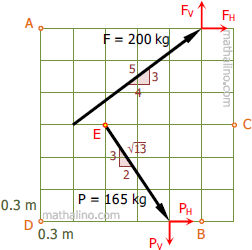
 FH=F(45)=200(45)
FH=F(45)=200(45)
FH=160 kg
FV=F(35)=200(35)
FV=120 kg
PH=P(2√13)=165(2√13)
PH=91.526 kg
PV=P(3√13)=165(3√13)
PV=137.288 kg
Moment of force F about points A, B, C, and D:
MA=5(0.3)FV=5(0.3)(120)
MA=180 kg⋅m → answer
MB=−6(0.3)FH=−6(0.3)(160)
MB=−288 kg⋅m → answer
MC=−0.3FV−3(0.3)FH=−0.3(120)−3(0.3)(160)
MC=−180 kg⋅m → answer
MD=5(0.3)FV−6(0.3)FH=5(0.3)(120)−6(0.3)(160)
MC=−108 kg⋅m → answer
Moment of force P about points A, B, C, and D:
MA=6(0.3)PH−4(0.3)PV=6(0.3)(91.526)−4(0.3)(137.288)
MA=0 (this means that point A is on the line of action of force P) → answer
MB=0.3PV=0.3(137.288)
MB=41.186 kg⋅m → answer
MC=2(0.3)PV+3(0.3)PH=2(0.3)(137.288)+3(0.3)(91.526)
MC=164.746 kg⋅m → answer
You can also resolve P to horizontal and vertical components at point E then take the moment of these components at point C. The answer would be the same. Try it.
MD=−4(0.3)PV=−4(0.3)(137.288)
MD=−164.746 kg⋅m → answer
