013 Resultant of three forces with angles greater than 90 degree

$R_x = 100 \cos 37^\circ + 100 \cos 150^\circ + 80 \cos 233^\circ$

$R_x = -54.88 \, \text{ N}$

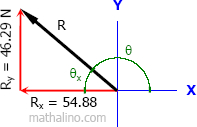
$R_x = 54.88 \, \text{ N to the left}$
 

 

$R_y = 100 \sin 37^\circ + 100 \sin 150^\circ + 80 \sin 233^\circ$

$R_y = 46.29 \, \text{ N}$
 

$R = \sqrt{{R_x}^2 + {R_y}^2}$

$R = \sqrt{54.88^2 + 46.29^2}$

$R = 71.79 \, \text{ N}$
 

$\tan \theta_x = \dfrac{R_y}{R_x}$

$\tan \theta_x = \dfrac{46.29}{54.88}$

$\theta_x = 40.15^\circ$
 

$\theta = 180^\circ - \theta_x = 180^\circ - 40.15^\circ$

$\theta = 139.85^\circ$
 

$R = 71.79 \, \text{ N at } \, 139.85^\circ$           answer