40 - Base angle of a growing right triangle

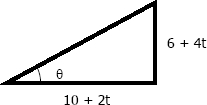
$\theta = \arctan \dfrac{6 + 4t}{10 + 2t}$

$\theta = \arctan \dfrac{3 + 2t}{5 + t}$

$\dfrac{d\theta}{dt} = \dfrac{\dfrac{(5 + t)(2) - (3 + 2t)(1)}{(5 + t)^2}}{1+ \left( \dfrac{3 + 2t}{5 + t} \right)^2}$

$\dfrac{d\theta}{dt} = \dfrac{\dfrac{10 + 2t - 3 - 2t}{(5 + t)^2}}{\dfrac{(5 + t)^2 + (3 + 2t)^2}{(5 + t)^2}}$

$\dfrac{d\theta}{dt} = \dfrac{7}{(5 + t)^2 + (3 + 2t)^2}$
 

when $\theta = 45^\circ$
$6 + 4t = 10 + 2t$

$2t = 4$

$t = 2 \, \text{ sec}$
 

Thus,
$\dfrac{d\theta}{dt} = \dfrac{7}{(5 + 2)^2 + [ \, 3 + 2(2) \, ]^2}$

$\dfrac{d\theta}{dt} = \dfrac{7}{49 + 49}$

$\dfrac{d\theta}{dt} = \dfrac{7}{98}$

$\dfrac{d\theta}{dt} = \dfrac{1}{14} \, \text{ rad/sec}$           answer

$\tan \theta = \dfrac{6 + 4t}{10 + 2t}$

$\tan \theta = \dfrac{3 + 2t}{5 + t}$

$\sec^2 \theta \dfrac{d\theta}{dt} = \dfrac{(5 + t)(2) - (3 + 2t)(1)}{(5 + t)^2}$

$\dfrac{1}{\cos^2 \theta} \times \dfrac{d\theta}{dt} = \dfrac{10 + 2t - 3 - 2t}{(5 + t)^2}$

$\dfrac{d\theta}{dt} = \cos^2 \theta \times \dfrac{7}{(5 + t)^2}$
 

when $\theta = 45^\circ$
$6 + 4t = 10 + 2t$

$2t = 4$

$t = 2 \, \text{ sec}$
 

Thus,
$\dfrac{d\theta}{dt} = \cos^2 45^\circ \times \dfrac{7}{(5 + 2)^2}$

$\dfrac{d\theta}{dt} = \left( \dfrac{1}{\sqrt{2}} \right)^2 \left( \dfrac{7}{7^2} \right)$

$\dfrac{d\theta}{dt} = \dfrac{1}{2} \times \dfrac{1}{7}$

$\dfrac{d\theta}{dt} = \dfrac{1}{14} \, \text{ rad/sec}$       (okay!)