258 Equivalent horizontal and vertical couples

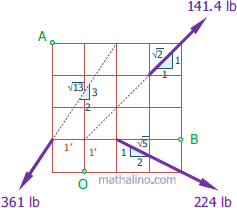
$R_x = \Sigma F_x$

$R_x = 141.4(\frac{1}{\sqrt{2}}) + 224(\frac{2}{\sqrt{5}}) - 361(\frac{2}{\sqrt{13}})$

$R_x = 100.09 \, \text{ lb to the right}$
 

$R_y = \Sigma F_y$

$R_y = 141.4(\frac{1}{\sqrt{2}}) - 224(\frac{1}{\sqrt{5}}) - 361(\frac{3}{\sqrt{13}})$

$R_y = -300.56 \, \text{ lb}$

$R_y = 300.56 \, \text{ lb downward}$
 

$R = \sqrt{{R_x}^2 + {R_y}^2}$

$R = \sqrt{100.09^2 + 300.56^2}$

$R = 316.79 \, \text{ lb downward to the right}$
 

$\tan \theta_x = \dfrac{R_y}{R_x}$

$\tan \theta_x = \dfrac{300.56}{100.09}$

$\theta_x = 71.58^\circ$
 

$M_O = -141.4(\frac{1}{\sqrt{2}})(1) - 224(\frac{1}{\sqrt{5}})(3) + 361(\frac{2}{\sqrt{13}})(1) + 361(\frac{3}{\sqrt{13}})(1)$

$M_O = 100.1 \text{ lb}\cdot\text{ft counterclockwise}$
 

Equivalent force through O and couple through A and B are shown in the figure below: