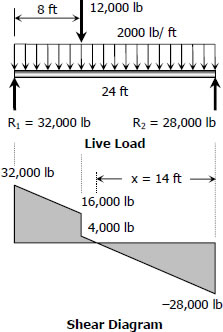
$\Sigma M_{R2} = 0$
$24R_1 = 16(12\,000) + 2000(24)(12)$
$R_1 = 32\,000 \, \text{lb}$
$\Sigma M_{R1} = 0$
$24R_2 = 8(12\,000) + 2000(24)(12)$
$R_2 = 28\,000 \, \text{lb}$
$\dfrac{x}{28\,000} = \dfrac{16 - x}{4000}$
$4000x = 28\,000(16 - x)$
$32\,000x = 448\,000$
$x = 14 \, \text{ft}$
$M_{live-load} = \frac{1}{2}x (28\,000) = \frac{1}{2}(14)(28\,000)$
$M_{live-load} = 196,000 \, \text{lb}\cdot\text{ft}$
$S_{required} \ge \dfrac{M_{live-load}}{(\,f_b\,)_{max}} \ge \dfrac{196\,000(12)}{18\,000}$
$S_{required} \ge 130.67 \, \text{in}^3$
From Appendix B, Table B-7 Properties of Wide-Flange Sections (W Shapes): US Customary Units, of text book:
| Designation |
Section Modulus |
| W12 × 96 |
131 in3 |
| W14 × 90 |
143 in3 |
| W16 × 77 |
134 in3 |
| W18 × 76 |
146 in3 |
| W21 × 68 |
140 in3 |
| W24 × 62 |
131 in3 |
Try W24 × 62 with S = 131 in3
Checking:
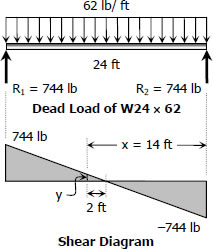$S_{resisting} \ge S_{live-load} + S_{dead-load}$
$S_{live-load} = 130.67 \, \text{in}^3$
$\dfrac{y}{2} = \dfrac{744}{12}$
$y = 124 \, \text{lb}$
At critical section:
$M_{dead-load} = \frac{1}{2}(744 + 124)(10)$
$M_{dead-load} = 4340 \, \text{lb}\cdot\text{ft}$
$S_{dead-load} = \dfrac{4340(12)}{18\,000} = 2.89 \, \text{in}^3$
$S_{live-load} + S_{dead-load} = 130.67 + 2.89$
$S_{live-load} + S_{dead-load} = 133.56 \, \text{in}^3$
$(S_{resisting} = 131 \, \text{in}^3) \lt 133.56 \, \text{in}^3$ (not okay!)
Try W21 × 68 with S = 140 in3
Checking:
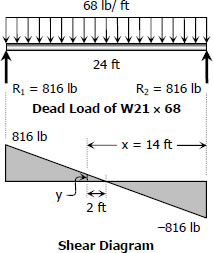$S_{resisting} \ge S_{live-load} + S_{dead-load}$
$S_{live-load} = 130.67 \, \text{in}^3$
$\dfrac{y}{2} = \dfrac{816}{12}$
$y = 136 \, \text{lb}$
At critical section:
$M_{dead-load} = \frac{1}{2}(816 + 136)(10)$
$M_{dead-load} = 4760 \, \text{lb}\cdot\text{ft}$
$S_{dead-load} = \dfrac{4760(12)}{18\,000} = 3.17 \, \text{in}^3$
$S_{live-load} + S_{dead-load} = 130.67 + 3.17$
$S_{live-load} + S_{dead-load} = 133.84 \,\text{in}^3$
$(S_{resisting} = 140 \, \text{in}^3) \gt 133.84 \, \text{in}^3$ (okay!)
Use W21 × 68 answer
Actual bending moment:
$M = M_{live-load} + M_{dead-load} = 196\,000 + 4\,760$
$M = 200\,760 \, \text{lb}\cdot\text{ft}$
Actual stress:
$(\,f_b\,)_{max} = \dfrac{M}{S} = \dfrac{200\,760(12)}{140}$
$(\,f_b\,)_{max} = 17\,208 \, \text{psi}$
$(\,f_b\,)_{max} = 17.208 \, \text{ksi}$ answer
