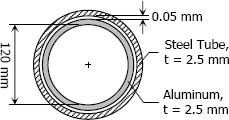
Internal pressure of aluminum tube to cause contact with the steel:
 δal=(σLE)al
δal=(σLE)al
π(122.6−122.5)=σ1(122.5π)70000
σ1=57.143MPa
p1D2t=57.143
p1(120)2(2.5)=57.143
p1=2.381 MPa → pressure that causes aluminum to contact with the steel, further increase of pressure will expand both aluminum and steel tubes.
Let pc = contact pressure between steel and aluminum tubes
2Pst+2Pal=F
2Pst+2Pal=5.0(120.1)(1)
Pst+Pal=300.25 → Equation (1)
The relationship of deformations is (from the figure):
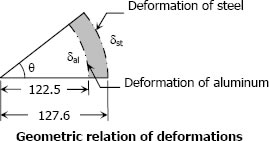 δst=127.6θ
δst=127.6θ
θ=δst/127.6
δal=122.5θ
δal=122.5(δst/127.6)
δal=0.96δst
(PLAE)al=0.96(PLAE)st
Pal(122.5π)2.5(70000)=0.96[Pst(127.6)2.5(200000)]
Pal=0.35Pst → Equation (2)
From Equation (1)
Pst+0.35Pst=300.25
Pst=222.41N
From Equation (2)
Pal=0.35(222.41)
Pal=77.84N
Contact Force
Fc+2Pst=F
pc(125.1)(1)+2(77.84)=5(120.1)(1)
pc=3.56 MPa answer

My question is how did the
My question is how did the modulus of elasticity (E) is 70000 in Al and 200000 in steel? Ty