The Regular Polygon

Rhombus is a quadrilateral with all sides equal (equilateral). Rectangle is a quadrilateral with all included angles are equal (equiangular). Square is both equilateral and equiangular, thus square is a regular polygon. Regular polygons are polygons with all sides equal and all included angles equal. Meaning, regular polygons are both equilateral and equiangular.

Properties of regular polygons

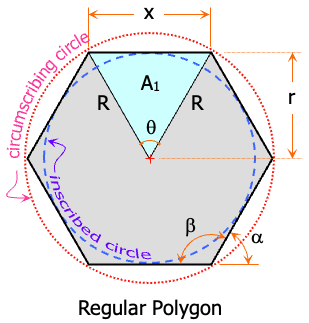
The center of the circumscribing circle, the center of inscribed circle, and the center of polygon itself are coincidence.
All sides of regular polygon are equal in length; it is denoted by x in the figure.
All included angles are equal; it is denoted by β.
All external angles α, are equal.
Central angles of each segment are equal; it is denoted by θ.
The apothem is the radius of the inscribed circle, r.
The number of sides is equal to the number of vertices, both are denoted by n.
Diagonals that pass through the center has length equal to the diameter of the circumscribing circle.
The triangular segment with area denoted as A₁ is an isosceles triangle. The length of the two equal sides of this triangle is the radius of the circumscribing circle and the altitude of this triangle is the radius of the inscribed circle.

Formulas for a Regular Polygon

Area of one segment, A₁

$A_1 = \frac{1}{2} xr$

$A_1 = \frac{1}{2}R^2 \sin \theta$

Total area, A

$A = nA_1$

$A = \dfrac{n}{2}xr$

$A = \dfrac{n}{2}R^2 \sin \theta$

Perimeter, P

$P = nx$

Central angle, θ

$\theta = \dfrac{360^\circ}{n}$

Exterior angle, α

$\alpha = \theta$

$\alpha = \dfrac{360^\circ}{n}$

Interior angle, β

$\beta = 180^\circ - \alpha$

$\beta = 180^\circ \left( \dfrac{n - 2}{n} \right)$

Where
A₁ = area of one segment
A = total area
x = length of side
r = radius of the inscribed circle (apothem)
R = radius of the circumscribing circle
n = number of sides
θ = central angle
α = exterior angle
β = interior angle

Navigation

Recent comments