Solution to Problem 516 | Flexure Formula

Problem 516
A timber beam AB, 6 in wide by 10 in deep and 10 ft long, is supported by a guy wire AC in the position shown in Fig. P-516. The beam carries a load, including its own weight, of 500 lb for each foot of its length. Compute the maximum flexural stress at the middle of the beam.
 

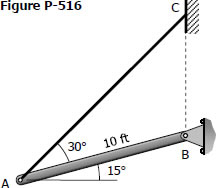
Solution 516
$x = 10 \cos 15^\circ$

$x = 9.66 \, \text{ft}$
 

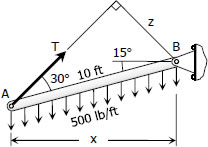$z = 10 \sin 30^\circ$

$z = 5 \, \text{ft}$
 

$\Sigma M_B = 0$

$zT = 500(10)(x/2)$

$5T = 500(10)(9.66/2)$

$T = 4829.63 \, \text{lb}$
 

At midspan:
$M = T(z/2) - 500(5)(x/4)$

$M = 4829.63(5/2) - 500(5)(9.66/4)$

$M = 6036.58 \, \text{lb}\cdot\text{ft}$
 

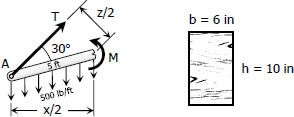
$f_b = \dfrac{Mc}{I} = \dfrac{M(h/2)}{\dfrac{bh^3}{12}}$

$f_b = \dfrac{6036.58(12)(10/2)}{\dfrac{6(10^3)}{12}}$

$f_b = 724.39 \, \text{psi}$           answer