Economic Sections

From the flexure formula $f_b = My / I$, it can be seen that the bending stress at the neutral axis, where $y = 0$, is zero and increases linearly outwards. This means that for a rectangular or circular section a large portion of the cross section near the middle section is understressed.
 

For steel beams or composite beams, instead of adopting the rectangular shape, the area may be arranged so as to give more area on the outer fiber and maintaining the same overall depth, and saving a lot of weight.
 

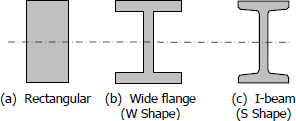
When using a wide flange or I-beam section for long beams, the compression flanges tend to buckle horizontally sidewise. This buckling is a column effect, which may be prevented by providing lateral support such as a floor system so that the full allowable stresses may be used, otherwise the stress should be reduced. The reduction of stresses for these beams will be discussed in steel design.
 

In selecting a structural section to be used as a beam, the resisting moment must be equal or greater than the applied bending moment. Note: $( f_b )_{max} = M/S$.
 

The equation above indicates that the required section modulus of the beam must be equal or greater than the ratio of bending moment to the maximum allowable stress.
 

A check that includes the weight of the selected beam is necessary to complete the calculation. In checking, the beams resisting moment must be equal or greater than the sum of the live-load moment caused by the applied loads and the dead-load moment caused by dead weight of the beam.
 

Dividing both sides of the above equation by $(f_b)_{max}$, we obtain the checking equation