Combined Axial and Bending

Combined axial compression and bending
$$\sigma = -\dfrac{P}{A} \pm \dfrac{Mc}{I}$$
 

Combined axial tension and bending
$$\sigma = \dfrac{P}{A} \pm \dfrac{Mc}{I}$$
 

For the flexure quantity $Mc/I$, use (+) for fibers in tension and (-) for fibers in compression.
 

Problem 902
Compare the maximum stress in bent rod 1/2 in. square, where the load P is 1/2 in. off center as shown in Figure P-902, with the maximum stress if the rod were straight and the load applied axially.
 

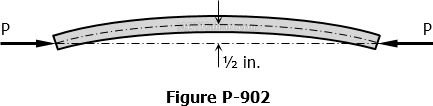
902-bar-in-eccentric-compression.gif

 

Solution 902
For P applied off-center:

The most stressed are the bottom fibers, it is subjected to compression due to axial load and compression due to bending.
$\sigma = -\dfrac{P}{A} - \dfrac{6M}{bd^2}$

$\sigma = -\dfrac{P}{0.5^2} - \dfrac{6(0.5P)}{0.5(0.5^2)}$

$\sigma = -28P$

 

For P applied axially:

$\sigma = -\dfrac{P}{A}$

$\sigma = -\dfrac{P}{0.5^2}$

$\sigma = -4P$

 

$\text{Ratio} = \dfrac{-28P}{-4P}$

$\text{Ratio} = \dfrac{7}{1}$       answer
 

Tags: 
Combined Stresses
Combined Axial and Bending