Flanged bolt couplings

In shaft connection called flanged bolt couplings (see figure), the torque is transmitted by the shearing force P created in he bolts that is assumed to be uniformly distributed. For any number of bolts n, the torque capacity of the coupling is
 

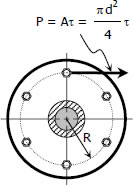
Flanged bolt couplings   Coupling bolts in one row

 

$T = PRn = \dfrac{\pi d^2}{4} \tau Rn$

 

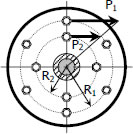
Coupling bolts in two rowsIf a coupling has two concentric rows of bolts, the torque capacity is
 

$T = P_1 R_1 n_1 + P_2 R_2 n_2$

 

where the subscript 1 refer to bolts on the outer circle an subscript 2 refer to bolts on the inner circle. See figure.
 

For rigid flanges, the shear deformations in the bolts are proportional to their radial distances from the shaft axis. The shearing strains are related by
 

$\dfrac{\gamma_1}{R_1} = \dfrac{\gamma_2}{R_2}$

 

Using Hooke's law for shear, G = τ / γ, we have
 

$\dfrac{\tau_1}{G_1 R_1} = \dfrac{\tau_2}{G_2 R_2}$   or   $\dfrac{P_1 / A_1}{G_1 R_1} = \dfrac{P_2 / A_2}{G_2 R_2}$

 

If the bolts on the two circles have the same area, A1 = A2, and if the bolts are made of the same material, G1 = G2, the relation between P1 and P2 reduces to
 

$\dfrac{P_1}{R_1} = \dfrac{P_2}{R_2}$

 

Tags
Torque
Torsional Shearing Stress
flanged bolt coupling