Active forum topics
- Hydraulics: Rotating Vessel
- Inverse Trigo
- General Solution of $y' = x \, \ln x$
- engineering economics: construct the cash flow diagram
- Eliminate the Arbitrary Constants
- Law of cosines
- Maxima and minima (trapezoidal gutter)
- Special products and factoring
- Integration of 4x^2/csc^3x√sinxcosx dx
- application of minima and maxima
New forum topics
- Hydraulics: Rotating Vessel
- Inverse Trigo
- General Solution of $y' = x \, \ln x$
- engineering economics: construct the cash flow diagram
- Integration of 4x^2/csc^3x√sinxcosx dx
- Maxima and minima (trapezoidal gutter)
- Special products and factoring
- Newton's Law of Cooling
- Law of cosines
- Can you help me po to solve this?
Recent comments
- Determine the least depth…1 week ago
- Solve mo ang h manually…2 weeks 4 days ago
- Paano kinuha yung height na…2 weeks 4 days ago
- It's the unit conversion…4 weeks 1 day ago
- Refer to the figure below…3 weeks 3 days ago
- Yes.4 months 2 weeks ago
- Sir what if we want to find…4 months 2 weeks ago
- Hello po! Question lang po…5 months ago
- 400000=120[14π(D2−10000)]
(…6 months 1 week ago - Use integration by parts for…7 months ago

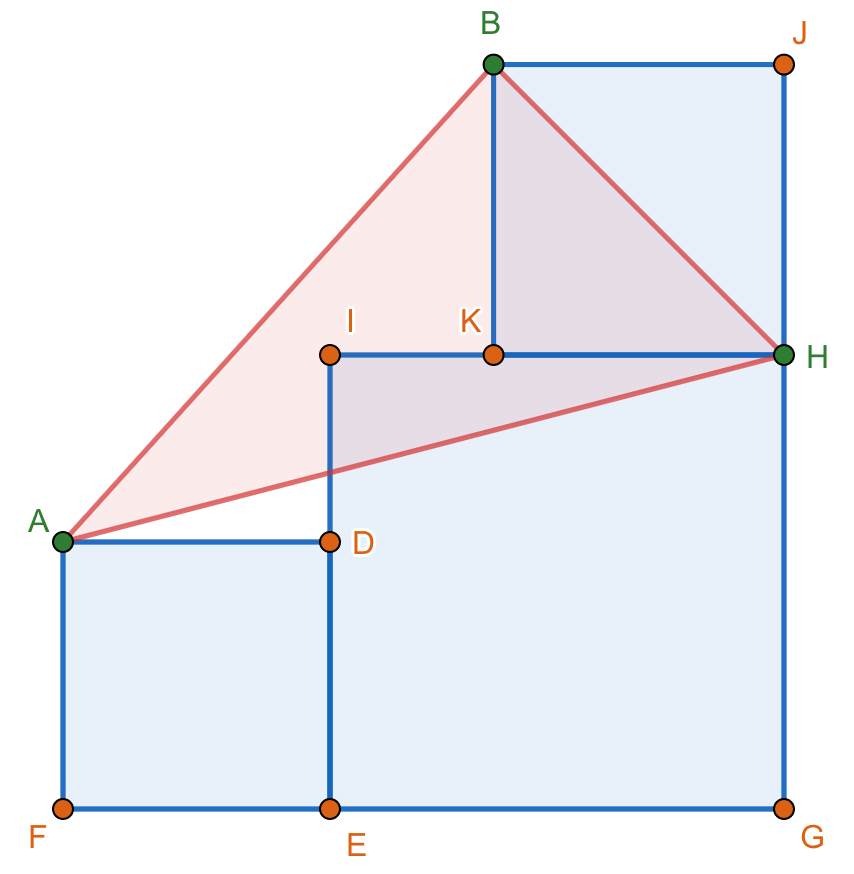
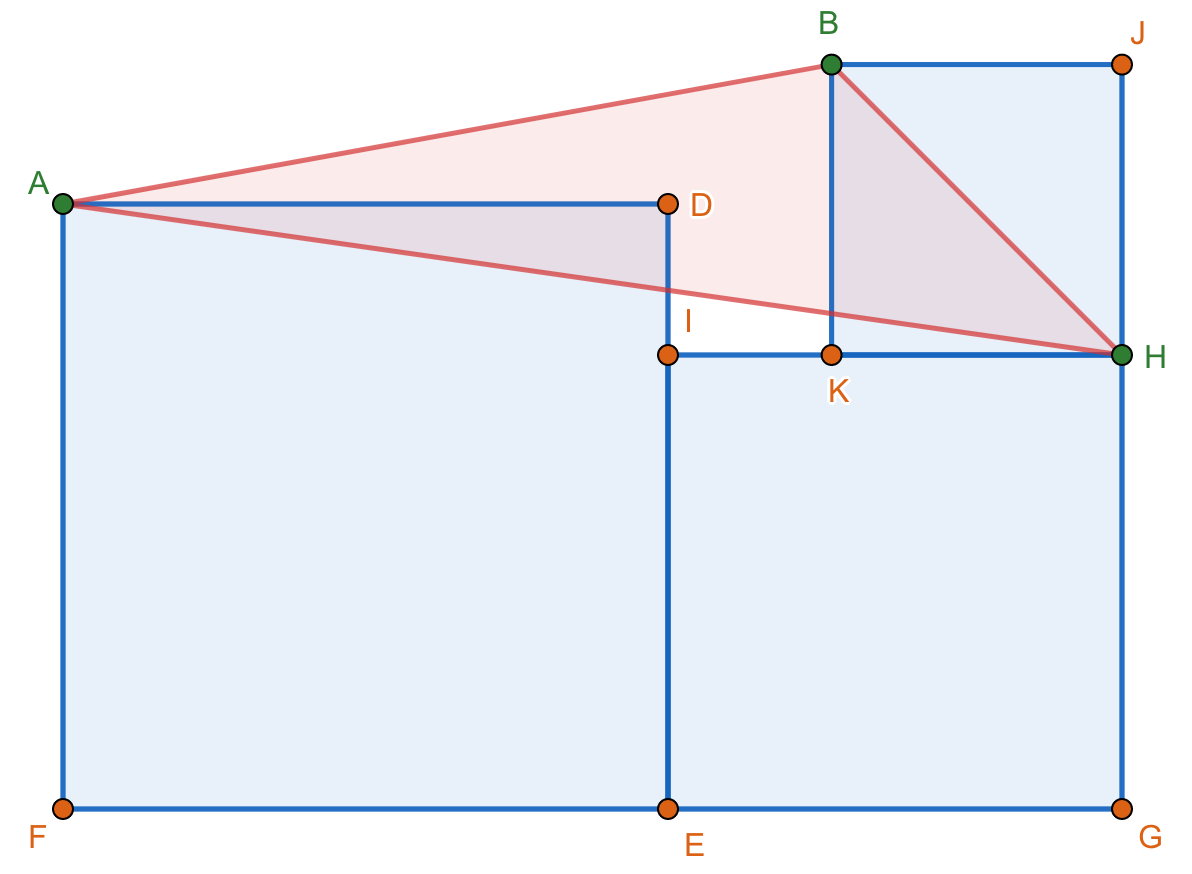

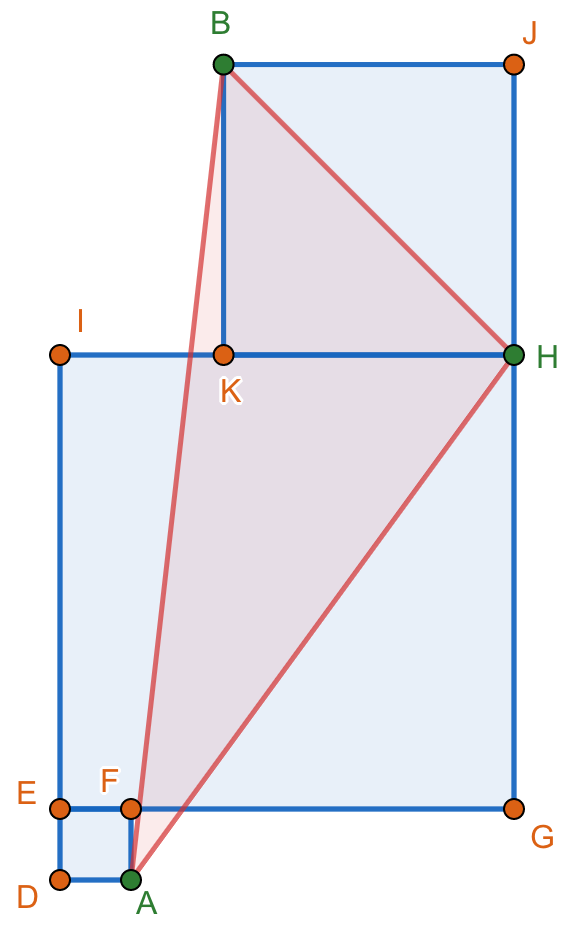

I don't really know how to
I don't really know how to prove this one sir. I just noticed when i substituted values, by taking line BH as the base, we will be having the same height in all triangles which we all know that if two triangles have the same base and height, their areas will be the same if i will not be mistaken.
Yes you are right. What you
In reply to I don't really know how to by MONGOL○482
Yes you are right. What you have in mind is the correct proof. All you need to do is to express it, the best is if you can articulate it through words and equations.
1. If we draw a line that
In reply to Yes you are right. What you by Jhun Vert
1. The diagonals of a square are perpendicular to each other.
2. If we draw a line extending diagonal AE of square AFED, we can see that vertex A is still going to land on this line no matter what the size of square AFED.
3. We can also see that diagonal AE is perpendicular to diagonal BH since their bases are parallel on the horizon lets say x.
Having these in mind and setting BH as the base of the triangle, and since vertex A can also land on vertex E, we can say that Area ABH=1/2 (BH)(EG2+GH2)1/2