cosine law | MATHalino reviewer about cosine law

Problem 10
In a triangle ABC, if $\dfrac{2 \cos A}{a} + \dfrac{\cos B}{b} + \dfrac{2 \cos C}{c} = \dfrac{a}{bc} + \dfrac{b}{ca}$ , find the value of angle $A$ .

Read more about Solving for angle $A$ in triangle ABC
Log in or register to post comments

Problem 13
The figure represents a rectangular parallelepiped; AD = 20 in., AB = 10 in., AE = 15 in.
(a) Find the number of degrees in the angles AFB, BFO, AFO, BOF, AOF, OFC.
(b) Find the area of each of the triangles ABO, BOF, AOF.
(c) Find the perpendicular distance from B to the plane AOF.

Area, angle, and distance in rectangular parallelepiped.

Solution 13

Read more about Solved Problem 13 | Rectangular Parallelepiped
Log in or register to post comments

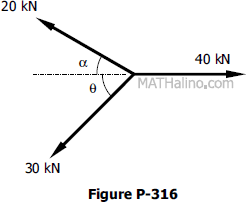
Problem 316
Determine the values of α and θ so that the forces shown in Fig. P-316 will be in equilibrium.

Read more about Problem 316 | Equilibrium of Concurrent Force System
Log in or register to post comments

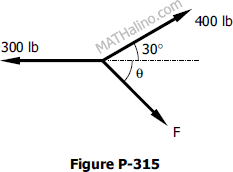
Problem 315
The 300-lb force and the 400-lb force shown in Fig. P-315 are to be held in equilibrium by a third force F acting at an unknown angle θ with the horizontal. Determine the values of F and θ.

Read more about Problem 315 | Equilibrium of Concurrent Force System
1 comment
Log in or register to post comments

For a cyclic quadrilateral with given sides a, b, c, and d, the formula for the area is given by

$A = \sqrt{(s - a)(s - b)(s - c)(s - d)}$

Where s = (a + b + c + d)/2 known as the semi-perimeter.

Read more about Derivation of Formula for Area of Cyclic Quadrilateral
Log in or register to post comments

Problem
A strip of 640 m² is sold from a triangular field whose sides are 96 m, 72 m, and 80 m. The strip is of uniform width h and has one of its sides parallel to the longest side of the field. Find the width of the strip.

A. 7.1 m
B. 8.1 m
C. 8.7 m
D. 7.7 m

Read more about Trapezoidal Strip of Land from a Triangular Lot
Log in or register to post comments

The following are the formulas for cosine law for any triangles with sides a, b, c and angles A, B, C, respectively.

$a^2 = b^2 + c^2 - 2bc\cos A$

$b^2 = a^2 + c^2 - 2ac\cos B$

$c^2 = a^2 + b^2 - 2ab\cos C$

Read more about Derivation of Cosine Law
Log in or register to post comments