The Polar Coordinate System

In Polar Coordinate System, the references are a fixed point and a fixed line. The fixed point is called the pole and the fixed line is called the polar axis. The location of a point is expressed according to its distance from the pole and its angle from the polar axis. The distance is denoted by r and the angle by θ.
 

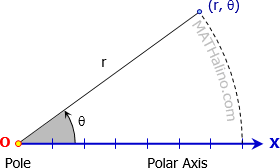
Plotting a Point in Polar Coordinate System

• θ is positive if measured counterclockwise
• θ is negative if measured clockwise
• r is positive if laid off at the terminal side of θ
• r is negative if laid off at the prolongation through O from the terminal side of θ

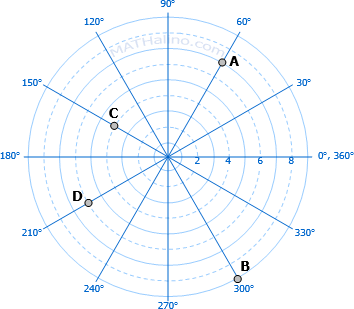
Example of point plotting
Plot the points A(3, 30°), B(5, -45°), C(-4, 75°), and D(-8, -60°) in polar coordinate system.
 

For easy plotting, identify the angle first by rotating appropriately from the polar axis. Counterclockwise for positive angle and clockwise for negative angle. Next is plot the distance. The point is on the terminal side of the angle if r is positive. If r is negative, the point is located on the other side of the pole along the line where the angle terminates.
 

From the figure above, point A is indicated by the angle blue, B is red, C is neon green, and D is in black color. Observe that the corresponding angle of each point is in the same color as the point.
 

Example of finding the polar coordinates of a point
Give the four basic polar coordinates of points A, B, C, and D shown in the figure. Basic polar coordinates are those coordinates with angles not lower than -360° and not higher than +360°.
 

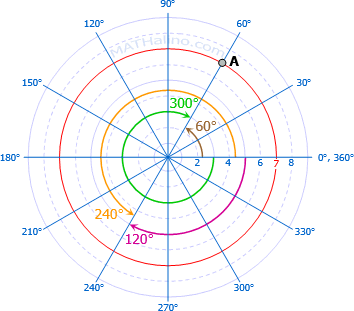Coordinates of point A: (7, 60°) (7, -300°) (-7, 240°) (-7, -120°)

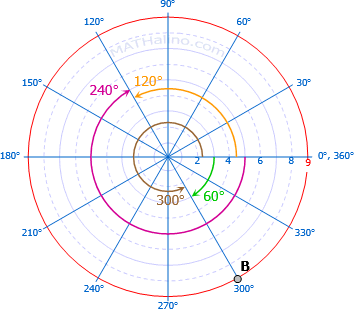Coordinates of point B: (9, 300°) (9, -60°) (-9, 120°) (-9, -240°)

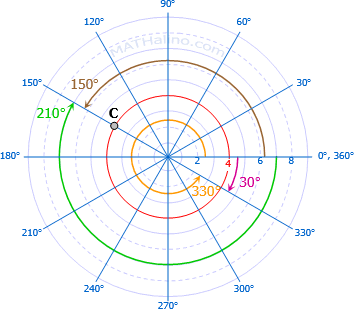Coordinates of point C: (4, 150°) (4, -210°) (-4, 330°) (-4, -30°)

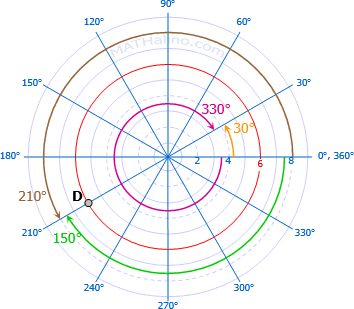Coordinates of point D: (6, 210°) (6, -150°) (-6, 30°) (-6, -330°)

Distance between Two Points in Polar Plane

The distance between two points (r₁, θ₁) and (r₂, θ₂) can be found using cosine law.
 

Relation between Polar and Rectangular Coordinate System

Equation in rectangular form may be converted to polar form or vice versa. The following relationships can be found from the figure shown.
 

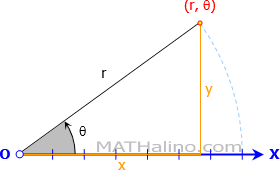
Polar to Rectangular
$x = r \cos \theta$

$y = r \sin \theta$
 

Rectangular to Polar
$r = \sqrt{x^2 + y^2}$

$\theta = \arctan \dfrac{y}{x}$
 

If you need a polar coordinate paper, you can download it here: https://mathalino.com/node/2347