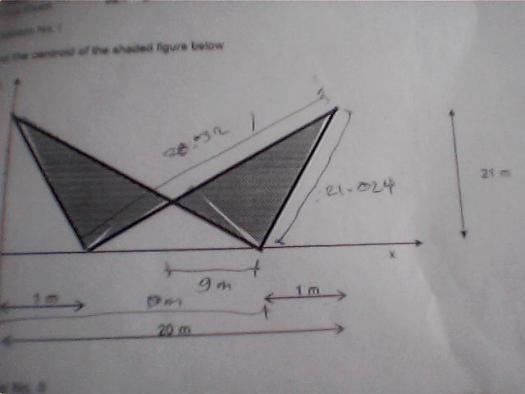
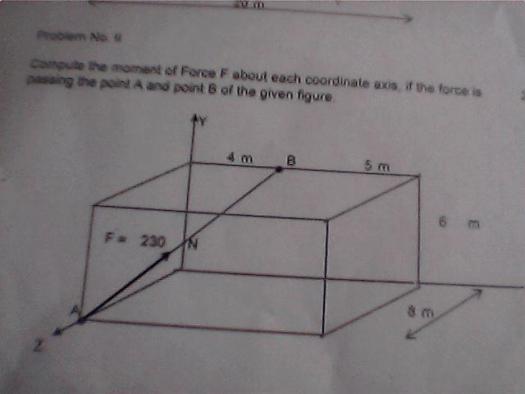
please help

What is ask in the first drawing?

For the second drawing:
$M_z = 0$   ←   Force F passes through the z-axis
 

$d = \sqrt{4^2 + 6^2 + 8^2}$

$d = 2\sqrt{29} ~ \text{m}$
 

$F_x = 230 \times \dfrac{4}{2\sqrt{29}} = 85.42 ~ \text{kN}$

$F_y = 230 \times \dfrac{6}{2\sqrt{29}} = 128.13 ~ \text{kN}$
 

$M_x = 8F_y = 1025.04 ~ \text{N}\cdot\text{m}$

$M_y = 8F_x = 683.36 ~ \text{N}\cdot\text{m}$

By symmetry
$\bar{x} = 10$
 

Equation of L1:
$\dfrac{x}{19} + \dfrac{y}{21} = 1$

$y = 21\left( 1 - \dfrac{x}{19} \right)$
 

At x = 10
$y = 21\left( 1 - \dfrac{10}{19} \right)$

$y = \frac{180}{19}$
 

$A_1 = 20(21) = 420$

$A_2 = 2 \times \frac{1}{2}(1)(21) = 21$

$A_3 = \frac{1}{2}(20)(21 - y) = \frac{2190}{19}$

$A_4 = \frac{1}{2}(18y) = \frac{1620}{19}$

$A = A_1 - A_2 - A_3 - A_4$
 

$y_1 = \frac{21}{2}$

$y_2 = \frac{21}{3}$

$y_3 = 21 - \frac{1}{3}(21 - y)$

$y_4 = \frac{1}{3}y$
 

$A\bar{y} = A_1 y_1 - A_2 y_2 - A_3 y_3 - A_4 y_4$ and solve for $\bar{y}$.
 

Centroid: $(10, \bar{y})$