Active forum topics
- Inverse Trigo
- General Solution of $y' = x \, \ln x$
- engineering economics: construct the cash flow diagram
- Eliminate the Arbitrary Constants
- Law of cosines
- Maxima and minima (trapezoidal gutter)
- Special products and factoring
- Integration of 4x^2/csc^3x√sinxcosx dx
- application of minima and maxima
- Sight Distance of Vertical Parabolic Curve
New forum topics
- Inverse Trigo
- General Solution of $y' = x \, \ln x$
- engineering economics: construct the cash flow diagram
- Integration of 4x^2/csc^3x√sinxcosx dx
- Maxima and minima (trapezoidal gutter)
- Special products and factoring
- Newton's Law of Cooling
- Law of cosines
- Can you help me po to solve this?
- Eliminate the Arbitrary Constants
Recent comments
- Yes.2 months ago
- Sir what if we want to find…2 months ago
- Hello po! Question lang po…2 months 2 weeks ago
- 400000=120[14π(D2−10000)]
(…3 months 3 weeks ago - Use integration by parts for…4 months 2 weeks ago
- need answer4 months 2 weeks ago
- Yes you are absolutely right…4 months 3 weeks ago
- I think what is ask is the…4 months 3 weeks ago
- $\cos \theta = \dfrac{2}{…4 months 3 weeks ago
- Why did you use (1/SQ root 5…4 months 3 weeks ago

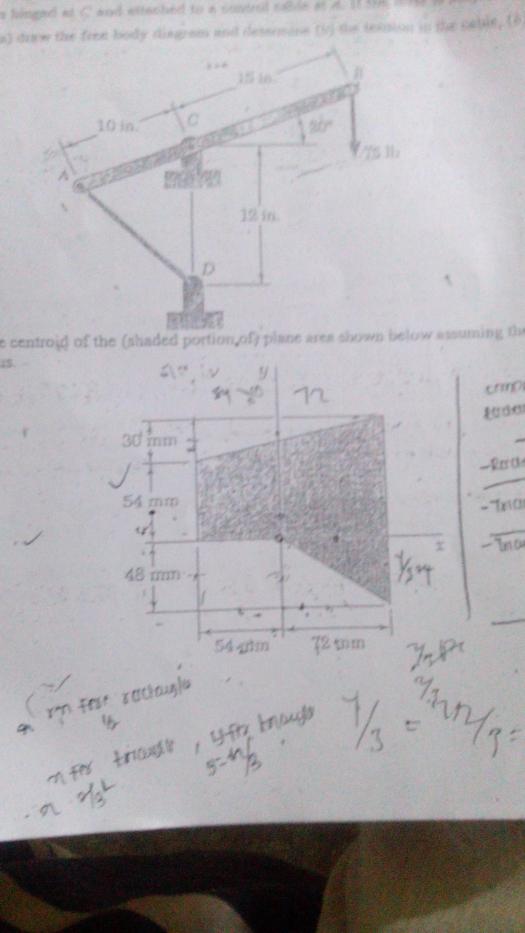 please
please
$A_1 = 126(54) = 6,804 ~
$A_1 = 126(54) = 6,804 ~ \text{mm}^2$
$x_1 = \frac{1}{2}(126) - 54 = 9 ~ \text{mm}$
$y_1 = \frac{1}{2}(54) = 27 ~ \text{mm}$
$A_2 = \frac{1}{2}(126)(30) = 1,890 ~ \text{mm}^2$
$x_2 = \frac{2}{3}(126) - 54 = 30 ~ \text{mm}$
$y_2 = 54 + \frac{1}{3}(30) = 64 ~ \text{mm}$
$A_3 = \frac{1}{2}(72)(48) = 1,728~ \text{mm}^2$
$x_3 = \frac{2}{3}(72) = 48 ~ \text{mm}$
$y_3 = -\frac{1}{3}(48) = -16 ~ \text{mm}$
$A = \Sigma A_n = 6,804 + 1,890 + 1,728$
$A = 10,422 ~ \text{mm}^2$
$\bar{x} = \dfrac{\Sigma A_n x_n}{A} = \dfrac{6804(9) + 1890(30) + 1728(48)}{10\,422}$
$\bar{y} = \dfrac{\Sigma A_n y_n}{A} = \dfrac{6804(27) + 1890(64) - 1728(16)}{10\,422}$