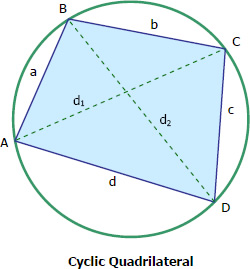
quadrilateral

For a cyclic quadrilateral with given sides a, b, c, and d, the formula for the area is given by
 

Where s = (a + b + c + d)/2 known as the semi-perimeter.
 

Problem PG-010
The quadrilateral ABCD shown in Fig. PG-010 is inscribed in a circle with side AD coinciding with the diameter of the circle. if sides AB, BC, and CD are 8 cm, 10 cm, and 12 cm long, respectively, find the radius of the circumscribing circle.
 

A quadrilateral is said to be cyclic if its vertices all lie on a circle. In cyclic quadrilateral, the sum of two opposite angles is 180° (or π radian); in other words, the two opposite angles are supplementary.

Quadrilateral is a polygon of four sides and four vertices. It is also called tetragon and quadrangle. In the triangle, the sum of the interior angles is 180°; for quadrilaterals the sum of the interior angles is always equal to 360°
 

Classifications of Quadrilaterals
There are two broad classifications of quadrilaterals; simple and complex. The sides of simple quadrilaterals do not cross each other while two sides of complex quadrilaterals cross each other.
 

Simple quadrilaterals are further classified into two: convex and concave. Convex if none of the sides pass through the quadrilateral when prolonged while concave if the prolongation of any one side will pass inside the quadrilateral.