Curvature and Radius of Curvature
Curvature (symbol, κ) is the mathematical expression of how much a curve actually curved. It is the measure of the average change in direction of the curve per unit of arc. Imagine a particle to move along the circle from point 1 to point 2, the higher the number of κ, the more quickly the particle changes in direction. This quick change in direction is apparent in smaller circles.
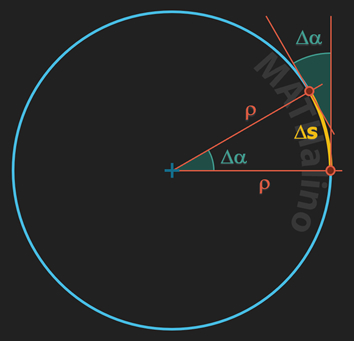
Δs=ρ⋅Δα
1ρ=ΔαΔs ← the curvature
Let 1/ρ = κ
κ=ΔαΔs
It is important to note that curvature κ is reciprocal to the radius of curvature ρ according to the above equations.

As P2 approaches P1, the ratio Δα/Δs approaches a limit. This limit is the curvature of the curve at a particular point, and from the above figure that point is P1.
κ=limΔs→0ΔαΔs
κ=dads
Curvature in xy-Plane
In a circle, κ is constant, however, if the curve in question is not a circle, κ represents the average curvature of the arc at a particular point.
From Analytic Geometry, the slope of the line m is equal to the tangent of angle of inclination, or m = tan α. Note that in Calculus, m = dy/dx.
tanα=y′
α=arctany′
Differentiate both sides with respect to x:
dα=y″dx1+(y′)2
Note that the Differential Length of Arc in the xy-plane is given by this formula:
ds=√1+(y′)2dx
Hence,
κ=dads=y″dx1+(y′)2√1+(y′)2dx
Simplify the equation above, and we have this formula for Curvature:
And for the Radius of Curvature:
