Constant Area of Triangle ABC
From the figures shown below. Squares EGHI and KHJB are fixed. Show that the area of triangle ABH is constant regardless of the dimensions of square ADEF.
Figure 1
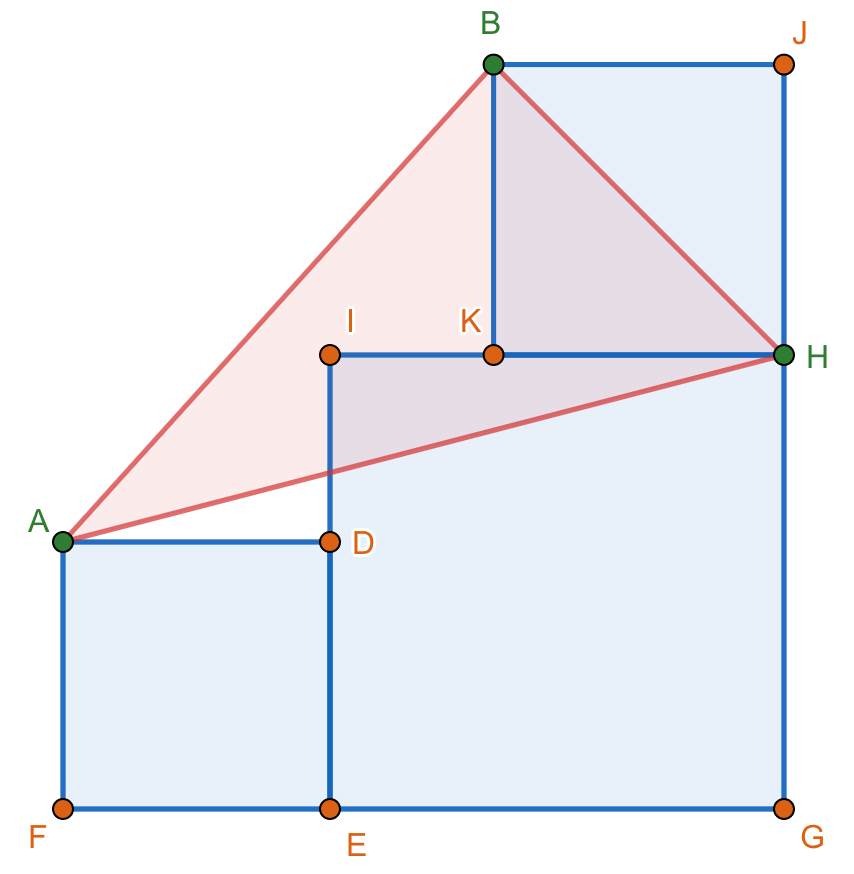
Figure 2
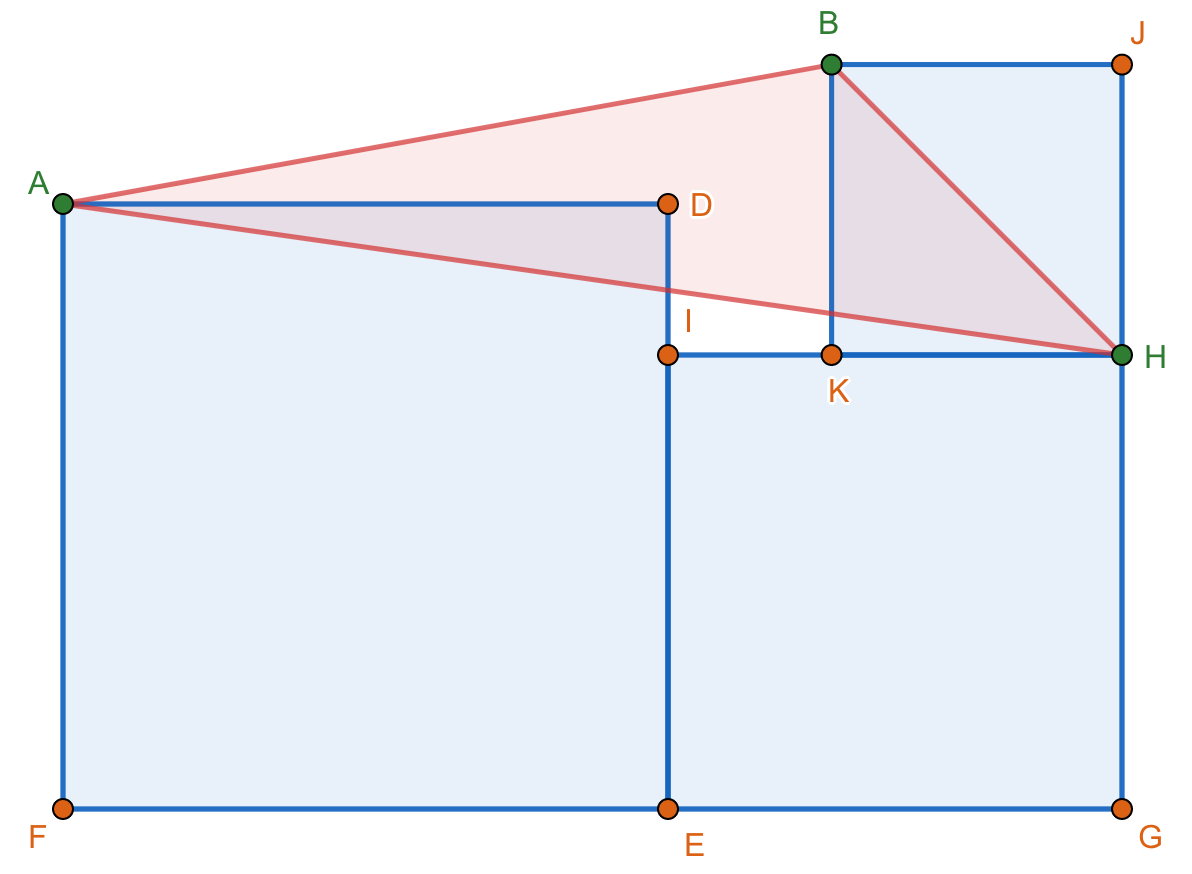
Figure 3

Figure 4
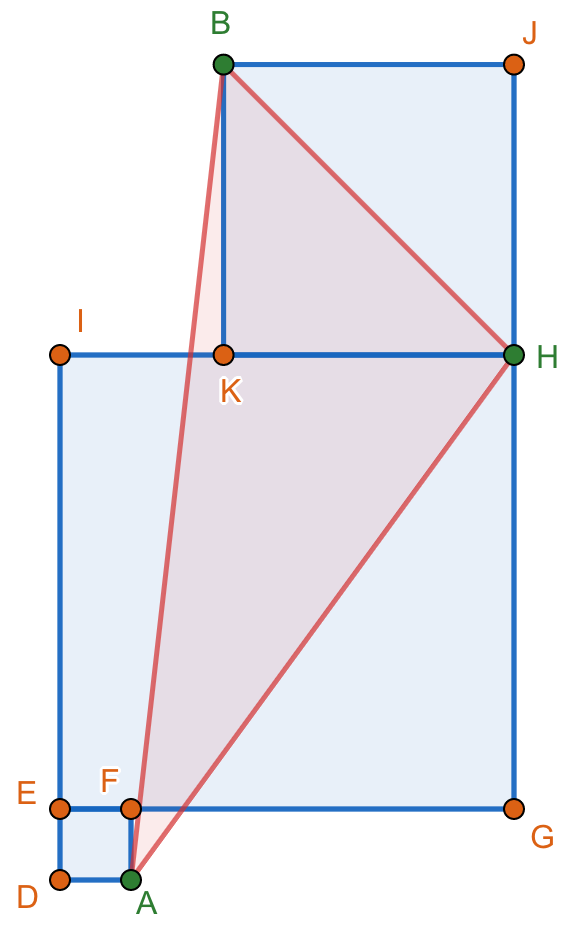
- Log in to post comments
Login here. Registration is temporarily disabled.
 MATHalinoEngineering Math Review
MATHalinoEngineering Math ReviewFrom the figures shown below. Squares EGHI and KHJB are fixed. Show that the area of triangle ABH is constant regardless of the dimensions of square ADEF.
Figure 1

Figure 2

Figure 3

Figure 4

I don't really know how to
Yes you are right. What you
In reply to I don't really know how to by MONGOL○482
Yes you are right. What you have in mind is the correct proof. All you need to do is to express it, the best is if you can articulate it through words and equations.
1. If we draw a line that
In reply to Yes you are right. What you by Jhun Vert