From the following figure, which of the following is equal to $\tan (\theta/2)$?
From the following figure, which of the following is equal to $\tan (\theta/2)$?
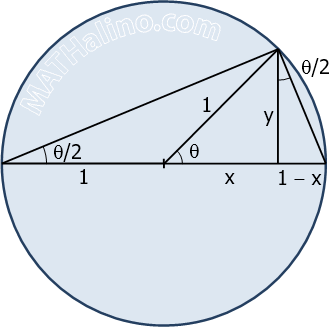
From the following figure, which of the following is equal to $\tan (\theta/2)$?

Solve for b as shown in the figure.
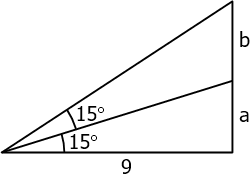
| A. $\sqrt{2} - 20$ | C. $12\sqrt{3} - 18$ |
| B. $6\sqrt{3} - 6$ | D. $4\sqrt{2} - 12$ |
If $\theta$ is an acute angle of a right triangle where $\sin \theta = x/2$, compute the value of $\sin 2\theta$ in terms of $x$.
| A. $\dfrac{x}{\sqrt{4 - x^2}}$ | C. $2x \sqrt{4 - x^2}$ |
| B. $\frac{1}{2}x \sqrt{4 - x^2}$ | D. $\dfrac{\sqrt{4 - x^2}}{x}$ |
Find $\tan \beta$ from the given figure.
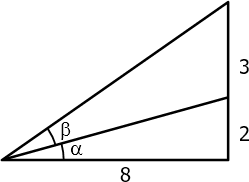
| A. 10/39 | C. 13/38 |
| B. 12/37 | D. 11/38 |
From the given right triangle what is the value of $3 \sin^2 \varphi \, \sec \varphi$ in terms of $x$.
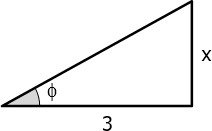
| A. $\dfrac{x}{\sqrt{x^2 + 9}}$ | C. $\dfrac{x^2 + 9}{\sqrt{x^2 - 9}}$ |
| B. $\dfrac{9}{\sqrt{x^2 + 9}}$ | D. $\dfrac{x^2}{\sqrt{x^2 + 9}}$ |
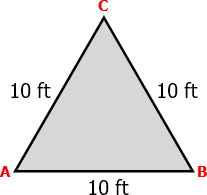
The equilateral triangle ABC represents a wooden platform standing in a lawn. A goat is tied to a corner with a 15 ft. rope. What is the maximum amount of grazing area available to the goat.
Find the area of the sector inside the square ABCD. Dimensions shown are in centimeter.
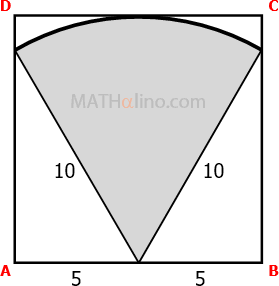
| A. 52.36 cm2 | C. 48.32 cm2 |
| B. 64.36 cm2 | D. 32.94 cm2 |
Find the value of $x = \sin^2 \varphi - \cos^2 \varphi$ if $\varphi$ is $\pi / 4$ or $45^\circ$.
| A. 3 | C. 1 |
| B. 2 | D. 0 |
Which of the following is equal to $\dfrac{\sin \theta}{1 + \cos \theta}$.
| A. $\dfrac{\sin \theta}{2 \sin 2\theta}$ | C. $\dfrac{\sin \theta}{1 + \sin \theta}$ |
| B. $\dfrac{\cos \theta}{1 - \sin \theta}$ | D. $\dfrac{1 - \cos \theta}{\sin \theta}$ |
Find the value of x if $x = \dfrac{1 + \tan^2 \varphi}{\tan \varphi \, \csc^2 \varphi}$.
| A. $\sin \varphi$ | C. $\tan \varphi$ |
| B. $\cos \varphi$ | D. $\csc \varphi$ |