Find $\displaystyle \sum_{n = 1}^5 S_n$ where $S_n = 2/n$.
Find $\displaystyle \sum_{n = 1}^5 S_n$ where $S_n = 2/n$.
| A. 137 / 30 | C. 142 / 28 |
| B. 154 / 29 | D. 142 / 30 |
Find $\displaystyle \sum_{n = 1}^5 S_n$ where $S_n = 2/n$.
| A. 137 / 30 | C. 142 / 28 |
| B. 154 / 29 | D. 142 / 30 |
Find the 6th term of $1, ~ 2, ~ 5, ~ ... \, , ~ \frac{1}{2}(1 + 3^{n - 1})$.
| A. 111 | C. 124 |
| B. 136 | D. 122 |
Find the 8th term of the sequence $-3, ~ 4, ~ 5/3, ~ … \, , ~ \dfrac{n + 2}{2n - 3}$.
| A. 9/12 | C. 6/15 |
| B. 10/13 | D. 12/17 |
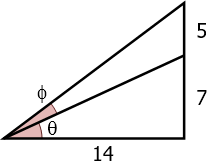
In the figure, $\varphi$ represents the angle subtended by a 5 ft picture when viewed from point P that is 7 ft below the picture and 14 ft away from the wall on which the picture hangs. Solve for $\varphi$ and express the answer using the arctan notation.
Solve for x if $\arcsin (x + 2) = \pi/6$.
| A. -3/2 | C. 3/4 |
| B. -2/3 | D. 3/8 |
Find the exact value of $\arccos (\tan 45^\circ)$.
| A. +1 | C. 0° |
| B. 2 | D. 45° |
Evaluate the $\tan (\arctan 3 + \arctan 4)$.
| A. 6/11 | C. 8/13 |
| B. -7/11 | D. -5/7 |
Evaluate the $\tan (\arctan 3 - \arctan 2)$.
| A. 1/2 | C. 3/8 |
| B. 2/3 | D. 1/7 |
Find the exact value of $\cos [ \, \arcsin (2/3) \, ]$.
| A. $\sqrt{5} / 2$ | C. $\sqrt{5} / 3$ |
| B. $3 / \sqrt{5}$ | D. $5 / \sqrt{5}$ |
At a point A that is 50 m from the base of a monument, the angle of elevation to the top of the monument is twice as large as the angle of elevation from a point B that is 150 m from the monument. Assuming that the base of the monument and the points A and B are in the same line on level ground, find the height of the monument.