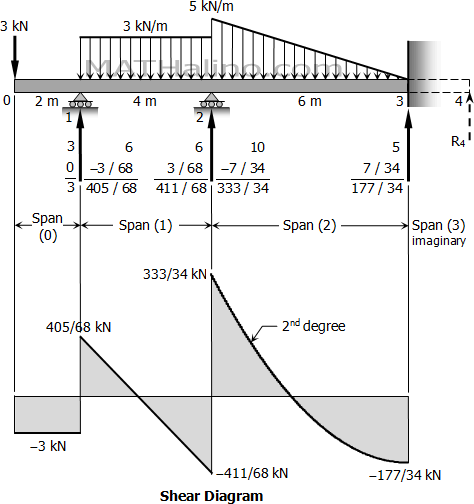
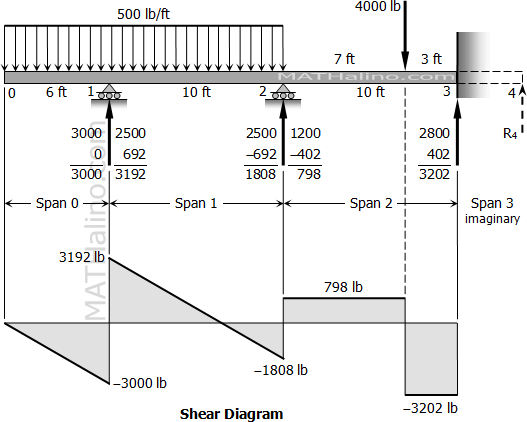
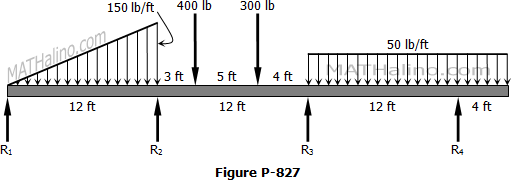
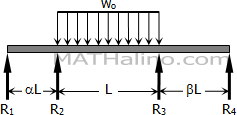
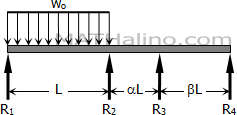
rectangular load

Problem 847
Compute the moments over the supports and sketch the shear diagram for the continuous beam shown in Fig. P-847.
 

Problem 846
Sketch the shear diagram for the continuous beam shown in Fig. P-846.
 

Problem 845
Compute the moments over the supports for the beam shown in Fig. P-845 and then draw the shear diagram.
 

Problem 827
See Figure P-827.
 

Problem 822
Solve Prob. 821 if the concentrated load is replaced by a uniformly distributed load of intensity w_o over the middle span.
 

Answers:
$M_2 = -\dfrac{w_o L^2}{4} \cdot \dfrac{1 + 2\beta}{4(\alpha + 1)(1 + \beta) - 1}$

$M_3 = -\dfrac{w_o L^2}{4} \cdot \dfrac{1 + 2\alpha}{4(1 + \alpha)(1 + \beta) - 1}$
 

Problem 820
Solve Prob. 819 if the concentrated load is replaced by a uniformly distributed load of intensity w_o over the first span.
 

Problem 334
Determine the reactions for the beam loaded as shown in Fig. P-334.
 

Problem 691
Determine the midspan deflection for the beam shown in Fig. P-691. (Hint: Apply Case No. 7 and integrate.)
 

Problem 689
The beam shown in Fig. P-689 has a rectangular cross section 4 inches wide by 8 inches deep. Compute the value of P that will limit the midspan deflection to 0.5 inch. Use E = 1.5 × 10⁶ psi.

Problem 687
Determine the midspan deflection of the beam shown in Fig. P-687 if E = 10 GPa and I = 20 × 10⁶ mm⁴.