midspan deflection
Solution to Problem 675 | Midspan Deflection
Problem 675
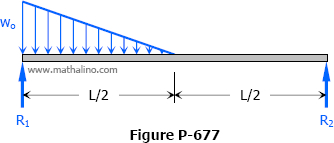
Repeat Prob. 674 for the overhanging beam shown in Fig. P-675.
- Read more about Solution to Problem 675 | Midspan Deflection
- Log in or register to post comments
Solution to Problem 674 | Midspan Deflection
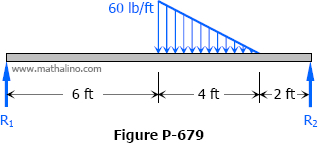
Problem 674
Find the deflection midway between the supports for the overhanging beam shown in Fig. P-674.

- Read more about Solution to Problem 674 | Midspan Deflection
- Log in or register to post comments
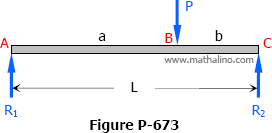
Solution to Problem 673 | Midspan Deflection
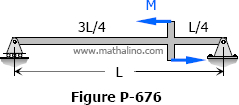
Problem 673
For the beam shown in Fig. P-673, show that the midspan deflection is δ = (Pb/48EI) (3L2 - 4b2).
- Read more about Solution to Problem 673 | Midspan Deflection
- Log in or register to post comments
Midspan Deflection | Deflections in Simply Supported Beams
In simply supported beams, the tangent drawn to the elastic curve at the point of maximum deflection is horizontal and parallel to the unloaded beam. It simply means that the deviation from unsettling supports to the horizontal tangent is equal to the maximum deflection. If the simple beam is symmetrically loaded, the maximum deflection will occur at the midspan.
Solution to Problem 665 | Deflections in Simply Supported Beams
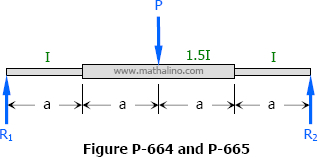
Problem 665
Replace the concentrated load in Prob. 664 by a uniformly distributed load of intensity wo acting over the middle half of the beam. Find the maximum deflection.
Solution to Problem 664 | Deflections in Simply Supported Beams
Problem 664
The middle half of the beam shown in Fig. P-664 has a moment of inertia 1.5 times that of the rest of the beam. Find the midspan deflection. (Hint: Convert the M diagram into an M/EI diagram.)