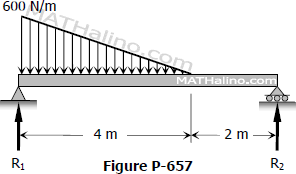
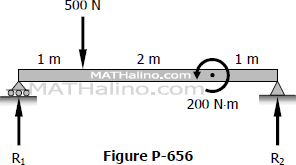
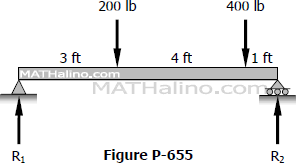
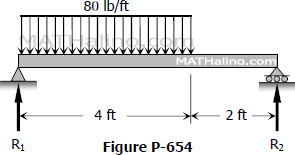
Problem 658 | Beam Deflection by Conjugate Beam Method
Problem 658
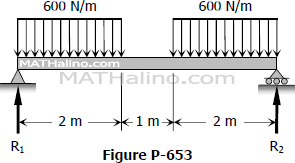
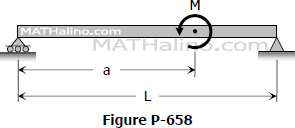
For the beam shown in Fig. P-658, find the value of EIδ at the point of application of the couple.
- Read more about Problem 658 | Beam Deflection by Conjugate Beam Method
- Log in or register to post comments