centroid
714 Inverted T-section | Centroid of Composite Figure
Problem 714
The dimensions of the T-section of a cast-iron beam are shown in Fig. P-714. How far is the centroid of the area above the base?

- Read more about 714 Inverted T-section | Centroid of Composite Figure
- Log in or register to post comments
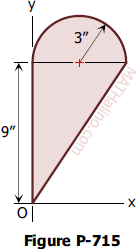
709 Centroid of the area bounded by one arc of sine curve and the x-axis
Problem 709
Locate the centroid of the area bounded by the x-axis and the sine curve $y = a \sin \dfrac{\pi x}{L}$ from x = 0 to x = L.
708 Centroid and area of spandrel by integration
Problem 708
Compute the area of the spandrel in Fig. P-708 bounded by the x-axis, the line x = b, and the curve y = kxn where n ≥ 0. What is the location of its centroid from the line x = b? Determine also the y coordinate of the centroid.

- Read more about 708 Centroid and area of spandrel by integration
- Log in or register to post comments
707 Centroid of quarter ellipse by integration
Problem 707
Determine the centroid of the quadrant of the ellipse shown in Fig. P-707. The equation of the ellipse is $\dfrac{x^2}{a^2} + \dfrac{y^2}{b^2} = 1$.

- Read more about 707 Centroid of quarter ellipse by integration
- Log in or register to post comments
706 Centroid of quarter circle by integration
Problem 706
Determine the centroid of the quarter circle shown in Fig. P-706 whose radius is r.

- Read more about 706 Centroid of quarter circle by integration
- Log in or register to post comments
705 Centroid of parabolic segment by integration
Problem 705
Determine the centroid of the shaded area shown in Fig. P-705, which is bounded by the x-axis, the line x = a and the parabola y2 = kx.

- Read more about 705 Centroid of parabolic segment by integration
- Log in or register to post comments
Centroids and Centers of Gravity
Centroids of Composite Figures
$W \, \bar{y} = \Sigma wy$
$A \, \bar{y} = \Sigma ay$
$L \, \bar{y} = \Sigma ly$
Back to top
Center of Gravity of Bodies and Centroids of Volumes
$W \, \bar{y} = \Sigma wy$
$W \, \bar{z} = \Sigma wz$
$V \, \bar{y} = \Sigma vy$
$V \, \bar{z} = \Sigma vz$
- Read more about Centroids and Centers of Gravity
- Log in or register to post comments
240 How to locate the centroid of metal plate with circular hole
Problem 240
The shaded area in Fig P-240 represents a steel plate of uniform thickness. A hole of 4-in. diameter has been cut in the plate. Locate the center of gravity the plate. Hint: The weight of the plate is equivalent to the weight of the original plate minus the weight of material cut away. Represent the original plate weight of plate by a downward force acting at the center of the 10 × 14 in. rectangle. Represent the weight of the material cut away by an upward force acting at the center of the circle. Locate the position of the resultant of these two forces with respect to the left edge and bottom of the plate.

Centers of a Triangle
This page will define the following: incenter, circumcenter, orthocenter, centroid, and Euler line.
Incenter
Incenter is the center of the inscribed circle (incircle) of the triangle, it is the point of intersection of the angle bisectors of the triangle.

The radius of incircle is given by the formula
where At = area of the triangle and s = ½ (a + b + c). See the derivation of formula for radius of incircle.
- Read more about Centers of a Triangle
- Log in or register to post comments