Solution to Problem 349 | Helical Springs
Problem 349
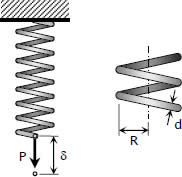
A rigid bar, hinged at one end, is supported by two identical springs as shown in Fig. P-349. Each spring consists of 20 turns of 10-mm wire having a mean diameter of 150 mm. Compute the maximum shearing stress in the springs, using Eq. (3-9). Neglect the mass of the rigid bar.
- Read more about Solution to Problem 349 | Helical Springs
- Log in or register to post comments