spherical triangle

Problem
An airplane flew from Davao City whose latitude is 14° N and longitude of 121.5° E on a course of S 30° W and maintaining uniform altitude. At what longitude will the plane cross the equator?
A. 110° 30' East
B. 122° 26' East
C. 113° 33' East
D. 116° 11' East
 

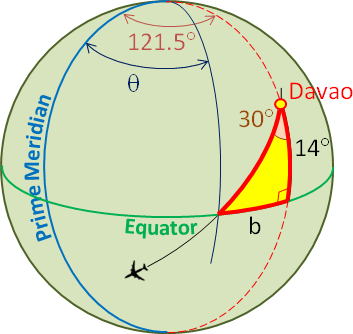
Problem
Find the area of a spherical triangle of whose angles are 123°, 84°, and 73°. The radius of the sphere is 30 m.

A. 1863.3 square meter
B. 1570.8 square meter
C. 1958.6 square meter
D. 1480.2 square meter
 

Definition of oblique spherical triangle
Spherical triangles are said to be oblique if none of its included angle is 90° or two or three of its included angles are 90°. Spherical triangle with only one included angle equal to 90° is a right triangle.
 

Sine law
$\dfrac{\sin a}{\sin A} = \dfrac{\sin b}{\sin B} = \dfrac{\sin c}{\sin C}$
 

Solution of right spherical triangle
With any two quantities given (three quantities if the right angle is counted), any right spherical triangle can be solved by following the Napier’s rules. The rules are aided with the Napier’s circle. In Napier’s circle, the sides and angle of the triangle are written in consecutive order (not including the right angle), and complimentary angles are taken for quantities opposite the right angle.
 

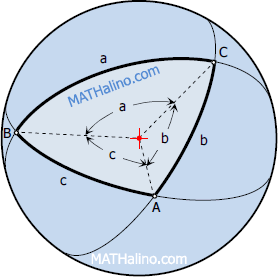
Spherical Triangle
Any section made by a cutting plane that passes through a sphere is circle. A great circle is formed when the cutting plane passes through the center of the sphere. Spherical triangle is a triangle bounded by arc of great circles of a sphere.