Uniformly Varying Load
Solution to Problem 670 | Deflections in Simply Supported Beams
Problem 670
Determine the value of EIδ at the left end of the overhanging beam shown in Fig. P-670.

Solution to Problem 657 | Deflections in Simply Supported Beams
Problem 657
Determine the midspan value of EIδ for the beam shown in Fig. P-657.

Resultant of Parallel Force System
Coplanar Parallel Force System
Parallel forces can be in the same or in opposite directions. The sign of the direction can be chosen arbitrarily, meaning, taking one direction as positive makes the opposite direction negative. The complete definition of the resultant is according to its magnitude, direction, and line of action.
- Read more about Resultant of Parallel Force System
- Log in or register to post comments
Solution to Problem 648 | Deflection of Cantilever Beams
Problem 648
For the cantilever beam loaded as shown in Fig. P-648, determine the deflection at a distance x from the support.

Solution to Problem 647 | Deflection of Cantilever Beams
Problem 647
Find the maximum value of EIδ for the beam shown in Fig. P-647.

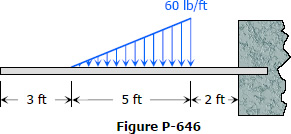
Solution to Problem 646 | Deflection of Cantilever Beams
Problem 646
For the beam shown in Fig. P-646, determine the value of I that will limit the maximum deflection to 0.50 in. Assume that E = 1.5 × 106 psi.
Solution to Problem 645 | Deflection of Cantilever Beams
Problem 645
Compute the deflection and slope at a section 3 m from the wall for the beam shown in Fig. P-645. Assume that E = 10 GPa and I = 30 × 106 mm4.